Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
2. Graphs of Equations
Lines
Problem 51b
Textbook Question
Write an equation (a) in standard form and (b) in slope-intercept form for each line described. through (-1, 4), parallel to x+3y=5
 Verified step by step guidance
Verified step by step guidance1
Identify the slope of the given line by rewriting the equation \(x + 3y = 5\) in slope-intercept form \(y = mx + b\).
Rewrite \(x + 3y = 5\) as \(3y = -x + 5\) and then divide by 3 to get \(y = -\frac{1}{3}x + \frac{5}{3}\).
Recognize that parallel lines have the same slope, so the slope of the new line is \(-\frac{1}{3}\).
Use the point-slope form \(y - y_1 = m(x - x_1)\) with point \((-1, 4)\) and slope \(-\frac{1}{3}\) to write the equation: \(y - 4 = -\frac{1}{3}(x + 1)\).
Convert the equation to standard form \(Ax + By = C\) by clearing fractions and rearranging terms.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Standard Form of a Linear Equation
The standard form of a linear equation is expressed as Ax + By = C, where A, B, and C are integers, and A should be non-negative. This form is useful for easily identifying the x- and y-intercepts of the line. To convert from slope-intercept form (y = mx + b) to standard form, you can rearrange the equation to fit the Ax + By = C format.
Recommended video:
Guided course
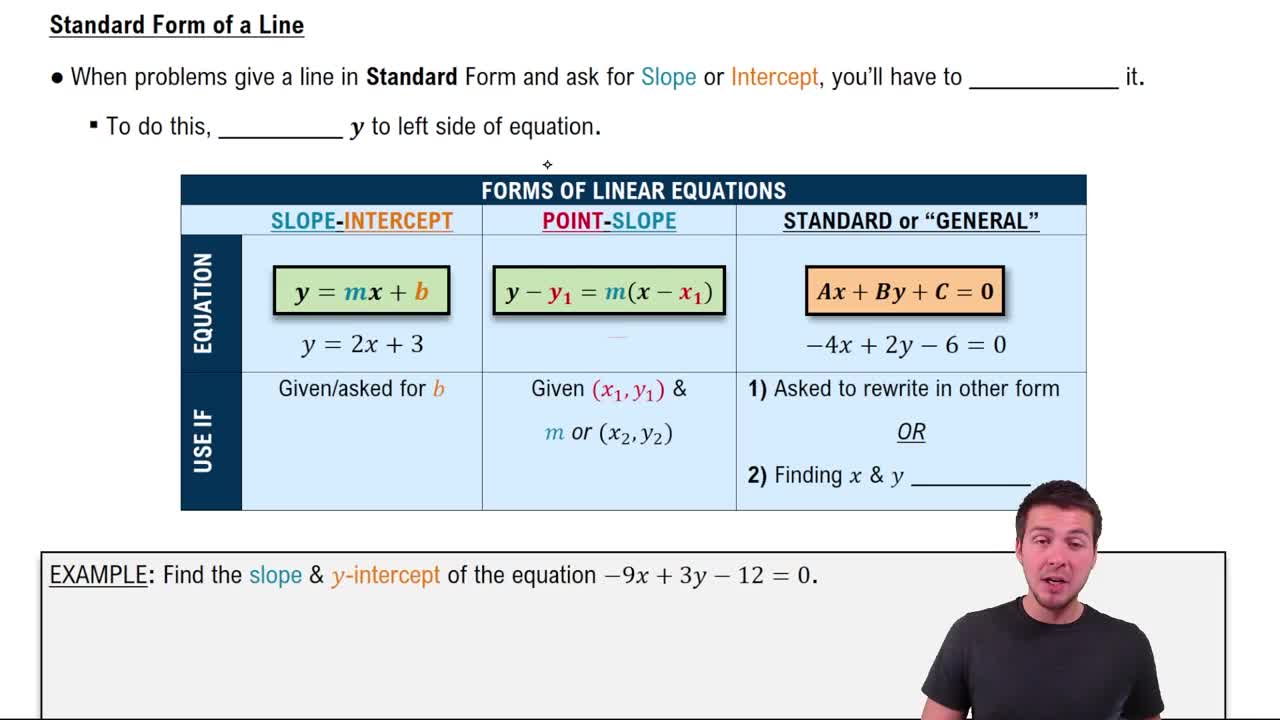
Standard Form of Line Equations
Slope-Intercept Form
The slope-intercept form of a linear equation is given by y = mx + b, where m represents the slope of the line and b is the y-intercept. This form is particularly useful for quickly graphing the line, as it directly provides the slope and where the line crosses the y-axis. Understanding how to manipulate this form is essential for solving problems involving linear equations.
Recommended video:
Guided course

Slope-Intercept Form
Parallel Lines
Two lines are parallel if they have the same slope but different y-intercepts. In the context of linear equations, if a line is parallel to another, it will not intersect it at any point. To find the equation of a line parallel to a given line, you can use the slope of the original line and the coordinates of a point through which the new line passes to derive its equation.
Recommended video:
Guided course

Parallel & Perpendicular Lines

 6:49m
6:49mWatch next
Master The Slope of a Line with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice









