Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
1. Equations & Inequalities
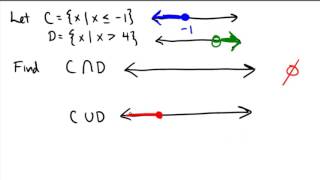
Linear Inequalities
Problem 4c
Textbook Question
Use the graph to solve each equation or inequality. Use interval
notation where appropriate. 7x(x - 1)(x - 2) < 0 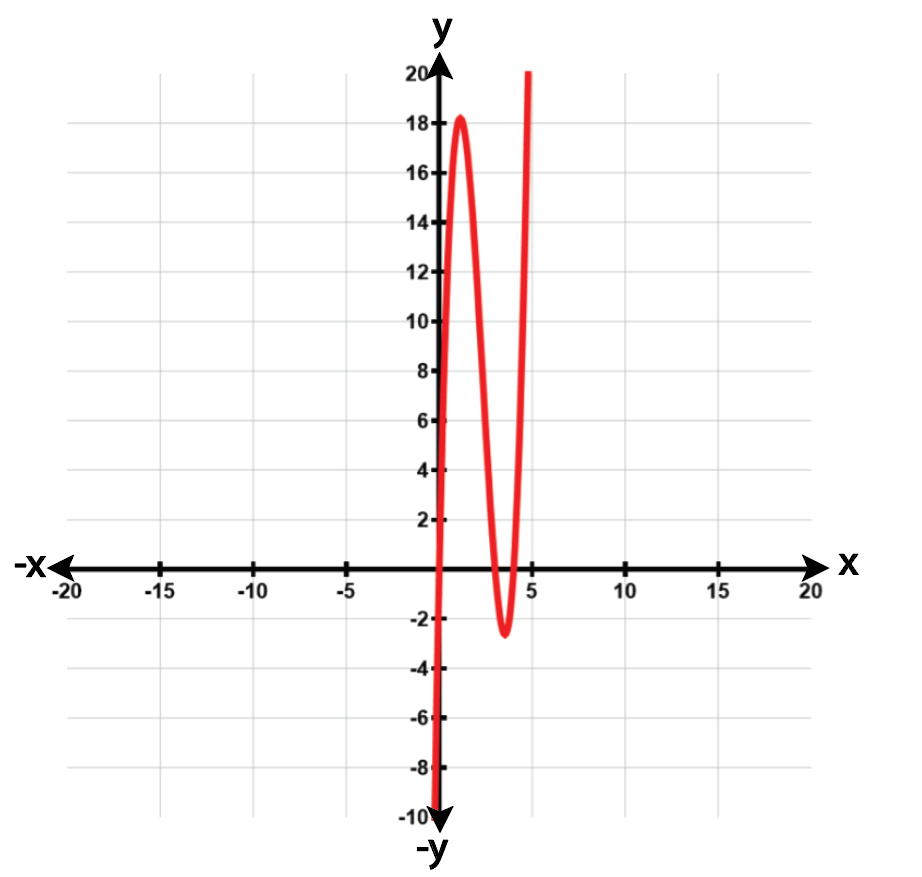
 Verified step by step guidance
Verified step by step guidance1
Identify the roots of the polynomial 7x(x - 1)(x - 2) by setting each factor equal to zero: x = 0, x = 1, and x = 2.
Plot these roots on the x-axis. These points divide the x-axis into four intervals: (-∞, 0), (0, 1), (1, 2), and (2, ∞).
Determine the sign of the polynomial in each interval by selecting a test point from each interval and substituting it into the polynomial.
Identify the intervals where the polynomial is less than zero based on the sign determined in the previous step.
Express the solution in interval notation, combining the intervals where the polynomial is less than zero.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
5mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Polynomial Functions
A polynomial function is a mathematical expression involving a sum of powers in one or more variables multiplied by coefficients. In this case, the function 7x(x - 1)(x - 2) is a cubic polynomial, which can have up to three real roots. Understanding the behavior of polynomial functions, including their roots and end behavior, is crucial for solving inequalities.
Recommended video:

Introduction to Polynomial Functions
Graphing Inequalities
Graphing inequalities involves determining the regions of the coordinate plane where the inequality holds true. For the inequality 7x(x - 1)(x - 2) < 0, we need to identify where the graph of the polynomial is below the x-axis. This requires analyzing the graph to find intervals where the function yields negative values.
Recommended video:
Guided course

Linear Inequalities
Interval Notation
Interval notation is a mathematical notation used to represent a range of values. It uses parentheses and brackets to indicate whether endpoints are included (closed interval) or excluded (open interval). For example, if the solution to the inequality is x in the interval (1, 2), it means x can take any value between 1 and 2, but not including 1 and 2 themselves.
Recommended video:

Interval Notation
Related Videos
Related Practice