Here are the essential concepts you must grasp in order to answer the question correctly.
Polynomial Multiplication
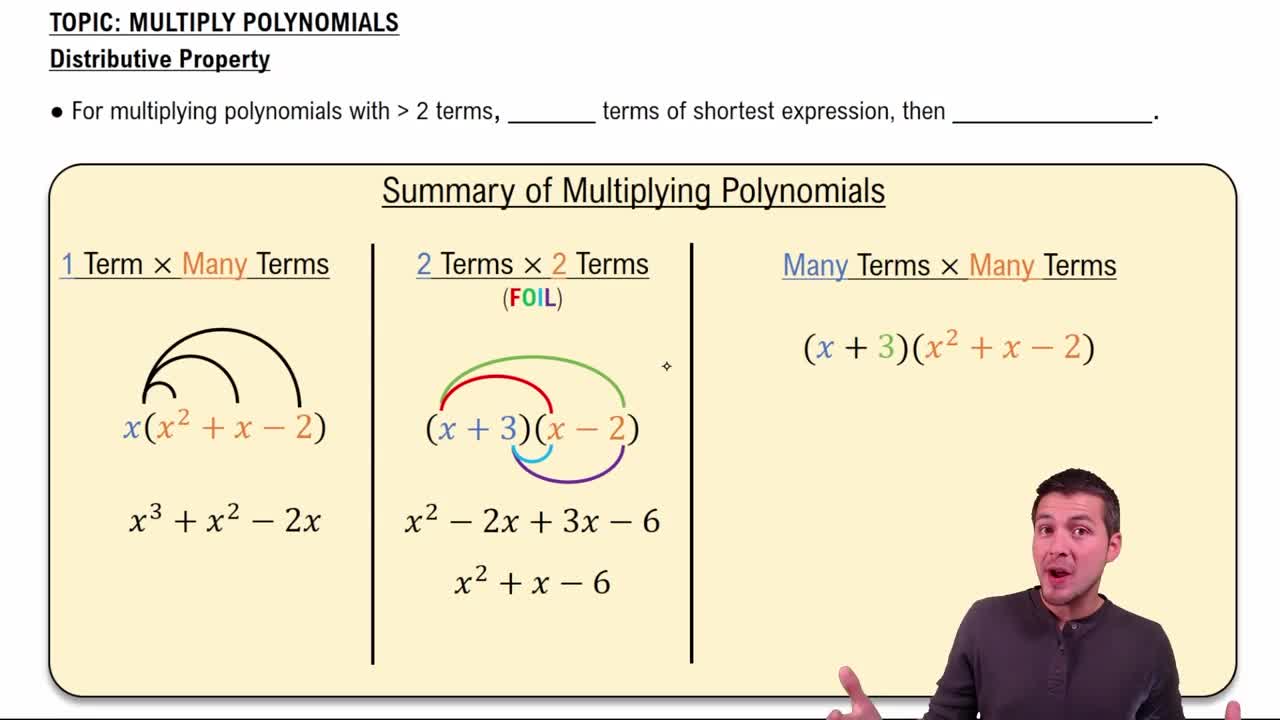
Polynomial multiplication involves distributing each term of one polynomial to every term of another polynomial. In this case, the expression (2x - 3) must be multiplied by each term in the polynomial (x^2 - 3x + 5). This process is often referred to as the distributive property and is essential for combining polynomials.
Recommended video:
Finding Zeros & Their Multiplicity
Distributive Property
The distributive property states that a(b + c) = ab + ac. This principle is crucial when multiplying polynomials, as it allows us to break down the multiplication into manageable parts. For the given expression, applying the distributive property will help in systematically calculating the product of the two polynomials.
Recommended video:
Multiply Polynomials Using the Distributive Property
Combining Like Terms
After multiplying the polynomials, the next step is to combine like terms, which are terms that have the same variable raised to the same power. This simplification is important for expressing the final result in its simplest form. In the context of the given problem, it ensures that the product is presented as a single polynomial rather than a sum of multiple terms.
Recommended video:
 Verified step by step guidance
Verified step by step guidance Verified Solution
Verified Solution


 5:13m
5:13m