Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
0. Review of Algebra
Multiplying Polynomials
Problem 9
Textbook Question
In Exercises 9–22, multiply the monomial and the polynomial. 4x²(3x+2)
 Verified step by step guidance
Verified step by step guidance1
Identify the monomial and the polynomial: The monomial is \(4x^2\) and the polynomial is \(3x + 2\).
Apply the distributive property: Multiply the monomial \(4x^2\) by each term in the polynomial \(3x + 2\).
First, multiply \(4x^2\) by \(3x\): Use the rule \(a^m \cdot a^n = a^{m+n}\) to combine the exponents of \(x\).
Next, multiply \(4x^2\) by \(2\): Simply multiply the coefficients and keep the \(x^2\) term as it is.
Combine the results from the previous steps to express the final expanded polynomial.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
1mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Monomials
A monomial is a mathematical expression that consists of a single term, which can be a number, a variable, or a product of numbers and variables raised to non-negative integer powers. In the given question, '4x²' is a monomial, representing a coefficient (4) multiplied by a variable (x) raised to the power of 2.
Recommended video:
Guided course

Introduction to Polynomials
Polynomials
A polynomial is an algebraic expression that consists of one or more terms, where each term is a product of a coefficient and variables raised to non-negative integer powers. The expression '3x + 2' is a polynomial with two terms, known as a binomial, which can be added or subtracted to form more complex expressions.
Recommended video:
Guided course

Introduction to Polynomials
Distributive Property
The distributive property is a fundamental algebraic principle that states a(b + c) = ab + ac. This property allows us to multiply a monomial by each term in a polynomial. In the exercise, applying the distributive property means multiplying '4x²' by both '3x' and '2' to find the resulting expression.
Recommended video:
Guided course
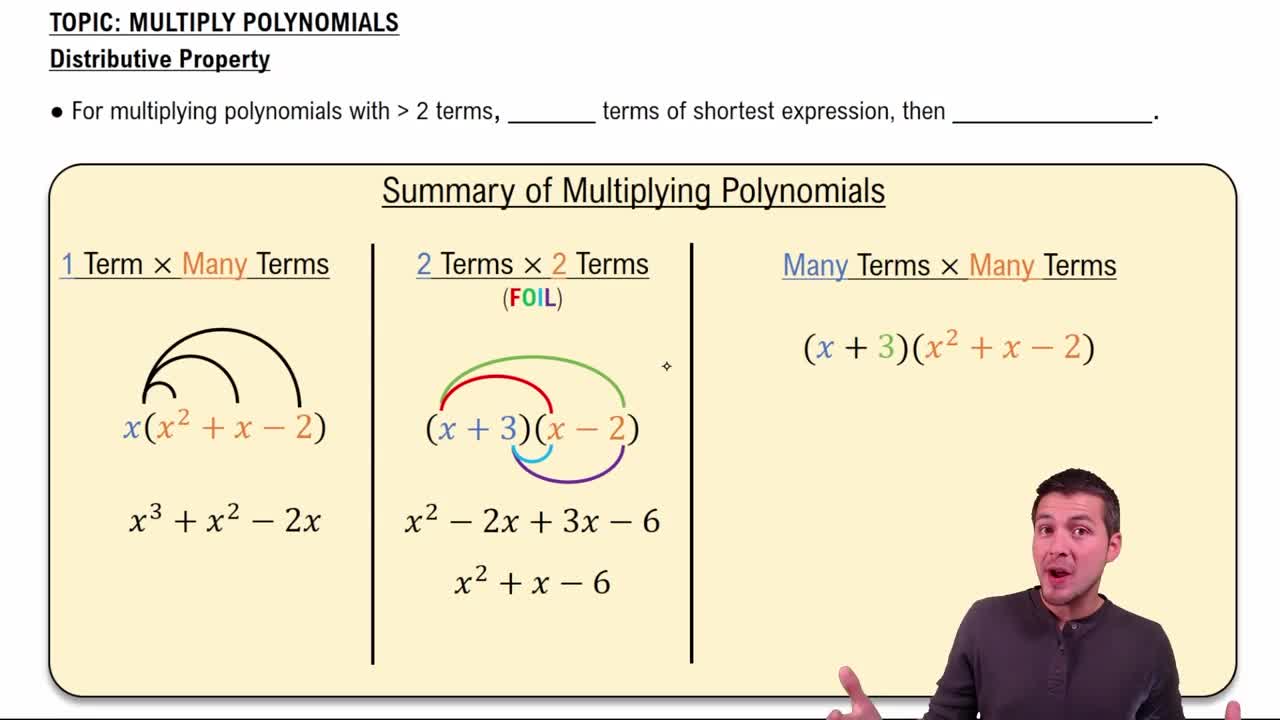
Multiply Polynomials Using the Distributive Property
Related Videos
Related Practice