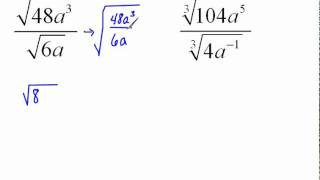In Exercises 1–20, use radical notation to rewrite each expression. Simplify, if possible. -16^¼

Verified step by step guidance

1. Identify the expression: \(-16^{\frac{1}{4}}\).
2. Recognize that the expression involves a negative base raised to a fractional exponent.
3. Rewrite the expression using radical notation: \(-16^{\frac{1}{4}} = \sqrt[4]{-16}\).
4. Understand that \(\sqrt[4]{-16}\) represents the fourth root of \(-16\).
5. Note that the fourth root of a negative number is not a real number, as even roots of negative numbers are not defined in the real number system.

Key Concepts

Radical Notation

Radical notation is a mathematical notation used to represent roots of numbers. The symbol '√' denotes the square root, while 'n√' represents the nth root of a number. For example, the expression 'x^(1/n)' can be rewritten as 'n√x', indicating the nth root of x. Understanding this notation is essential for rewriting expressions involving roots.

Exponents and Fractional Powers

Exponents are a way to express repeated multiplication of a number by itself. A fractional exponent, such as '1/4', indicates a root; specifically, 'x^(1/n)' means the nth root of x. In the case of '-16^(1/4)', it signifies the fourth root of -16, which is crucial for simplifying the expression correctly.

Simplifying Radical Expressions

Simplifying radical expressions involves reducing them to their simplest form, which often includes factoring out perfect squares or cubes. For instance, when simplifying '√(a*b)', one can separate it into '√a * √b'. In the context of '-16^(1/4)', recognizing that -16 can be expressed as '(-1) * (16)' helps in simplifying the expression further.