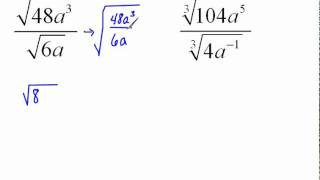Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
0. Review of Algebra
Radical Expressions
Problem 54b
Textbook Question
In Exercises 39–60, simplify by factoring. Assume that all variables in a radicand represent positive real numbers and no radicands involve negative quantities raised to even powers. ___ ⁵√y¹⁸
 Verified step by step guidance
Verified step by step guidance1
<insert step 1: Recognize that the expression involves a radical, specifically a fifth root, and an exponent inside the radical.>
<insert step 2: Recall the property of radicals and exponents: \( \sqrt[n]{a^m} = a^{m/n} \).>
<insert step 3: Apply this property to the given expression: \( \sqrt[5]{y^{18}} = y^{18/5} \).>
<insert step 4: Simplify the exponent \( \frac{18}{5} \) by dividing 18 by 5, which can be expressed as a mixed number or a decimal.>
<insert step 5: Express the result as a power of \( y \) with the simplified exponent.>
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
4mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Radicals
Radicals are expressions that involve roots, such as square roots, cube roots, and higher-order roots. In this context, the expression ⁵√y¹⁸ represents the fifth root of y raised to the 18th power. Understanding how to manipulate radicals is essential for simplifying expressions involving roots.
Recommended video:
Guided course

Expanding Radicals
Exponent Rules
Exponent rules govern how to simplify expressions involving powers. For instance, when taking the root of a power, the exponent can be divided by the root's index. In this case, y¹⁸ can be simplified using the rule that states ⁵√(y^n) = y^(n/5), which is crucial for simplifying the given expression.
Recommended video:
Guided course

Introduction to Exponent Rules
Factoring
Factoring is the process of breaking down an expression into simpler components, often to simplify or solve equations. In the context of radicals, factoring can help identify perfect powers that can be simplified further. Understanding how to factor expressions is key to simplifying the radical expression effectively.
Recommended video:
Guided course

Factor by Grouping
Related Videos
Related Practice