Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
7. Systems of Equations & Matrices
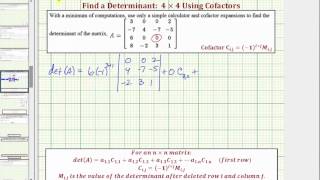
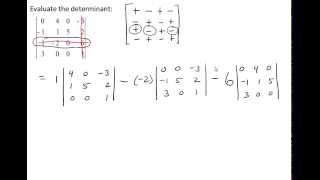
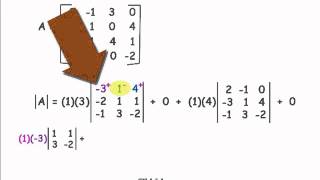
Determinants and Cramer's Rule
Problem 49a
Textbook Question
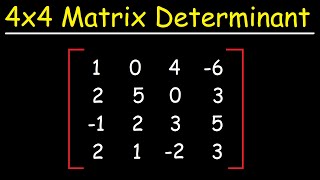
Evaluate each determinant in Exercises 49–52.
4 2 8 - 7
- 2 0 4 1
5 0 0 5
4 0 0 - 1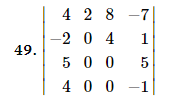
 Verified step by step guidance
Verified step by step guidance1
Identify the matrix as a 4x4 matrix.
Use the cofactor expansion method along the first row to evaluate the determinant.
Calculate the determinant of the 3x3 submatrix obtained by removing the first row and first column.
Repeat the process for the remaining elements of the first row, considering their respective 3x3 submatrices.
Sum the results, taking into account the alternating signs in the cofactor expansion.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
7mPlay a video:
Was this helpful?

 4:36m
4:36mWatch next
Master Determinants of 2×2 Matrices with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice