Here are the essential concepts you must grasp in order to answer the question correctly.
Completing the Square
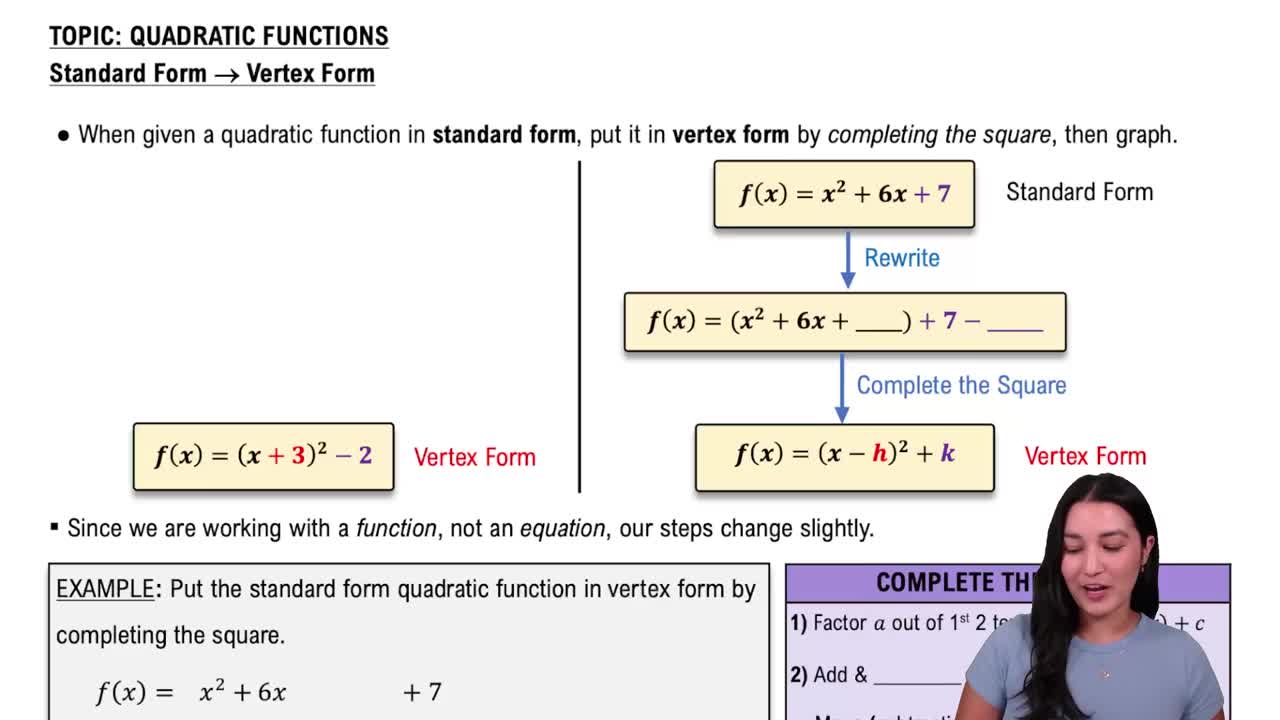
Completing the square is a method used to solve quadratic equations by transforming the equation into a perfect square trinomial. This involves rearranging the equation and adding a specific value to both sides to create a square of a binomial. This technique simplifies the process of finding the roots of the equation and is particularly useful when the quadratic is not easily factorable.
Recommended video:
Solving Quadratic Equations by Completing the Square
Quadratic Equations
A quadratic equation is a polynomial equation of the form ax^2 + bx + c = 0, where a, b, and c are constants, and a is not zero. The solutions to these equations can be found using various methods, including factoring, using the quadratic formula, or completing the square. Understanding the standard form of a quadratic equation is essential for applying these methods effectively.
Recommended video:
Introduction to Quadratic Equations
Standard Form of a Quadratic
The standard form of a quadratic equation is expressed as y = ax^2 + bx + c. This form allows for easy identification of the coefficients a, b, and c, which are crucial for determining the properties of the parabola represented by the equation, such as its vertex, axis of symmetry, and direction of opening. Recognizing this form is vital for applying techniques like completing the square.
Recommended video:
Converting Standard Form to Vertex Form
 Verified Solution
Verified Solution


 5:35m
5:35m
