Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
1. Equations & Inequalities
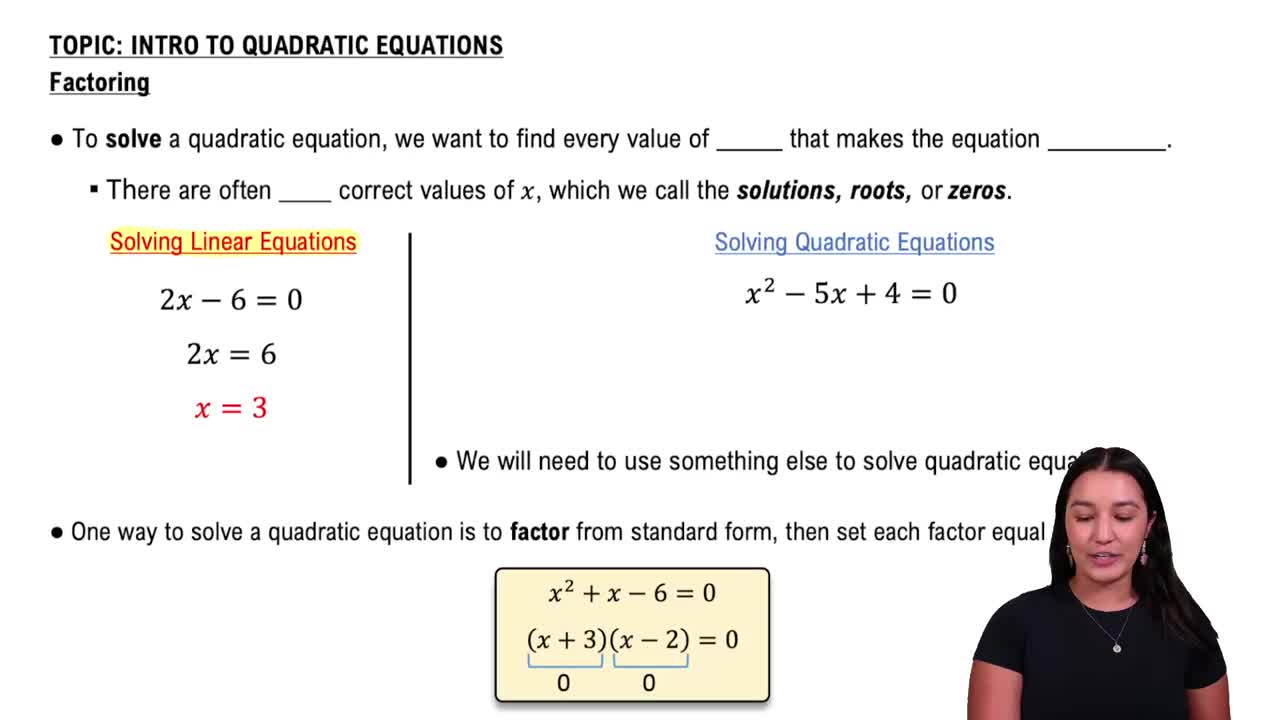
Intro to Quadratic Equations
Problem 43a
Textbook Question
Solve each equation using completing the square. See Examples 3 and 4. 2x^2 + x = 10
 Verified step by step guidance
Verified step by step guidance1
Start by moving the constant term to the other side of the equation: \(2x^2 + x - 10 = 0\).
Divide every term by the coefficient of \(x^2\), which is 2, to simplify: \(x^2 + \frac{1}{2}x = 5\).
To complete the square, take half of the coefficient of \(x\), which is \(\frac{1}{2}\), square it, and add it to both sides: \(x^2 + \frac{1}{2}x + \left(\frac{1}{4}\right)^2 = 5 + \left(\frac{1}{4}\right)^2\).
Rewrite the left side as a perfect square trinomial: \((x + \frac{1}{4})^2 = 5 + \frac{1}{16}\).
Solve for \(x\) by taking the square root of both sides and then isolating \(x\): \(x + \frac{1}{4} = \pm \sqrt{5 + \frac{1}{16}}\).
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Completing the Square
Completing the square is a method used to solve quadratic equations by transforming the equation into a perfect square trinomial. This involves rearranging the equation and adding a specific value to both sides to create a square of a binomial. This technique simplifies the process of finding the roots of the equation and is particularly useful when the quadratic is not easily factorable.
Recommended video:

Solving Quadratic Equations by Completing the Square
Quadratic Equations
A quadratic equation is a polynomial equation of the form ax^2 + bx + c = 0, where a, b, and c are constants, and a is not zero. The solutions to these equations can be found using various methods, including factoring, using the quadratic formula, or completing the square. Understanding the standard form of a quadratic equation is essential for applying these methods effectively.
Recommended video:

Introduction to Quadratic Equations
Standard Form of a Quadratic
The standard form of a quadratic equation is expressed as y = ax^2 + bx + c. This form allows for easy identification of the coefficients a, b, and c, which are crucial for determining the properties of the parabola represented by the equation, such as its vertex, axis of symmetry, and direction of opening. Recognizing this form is vital for applying techniques like completing the square.
Recommended video:
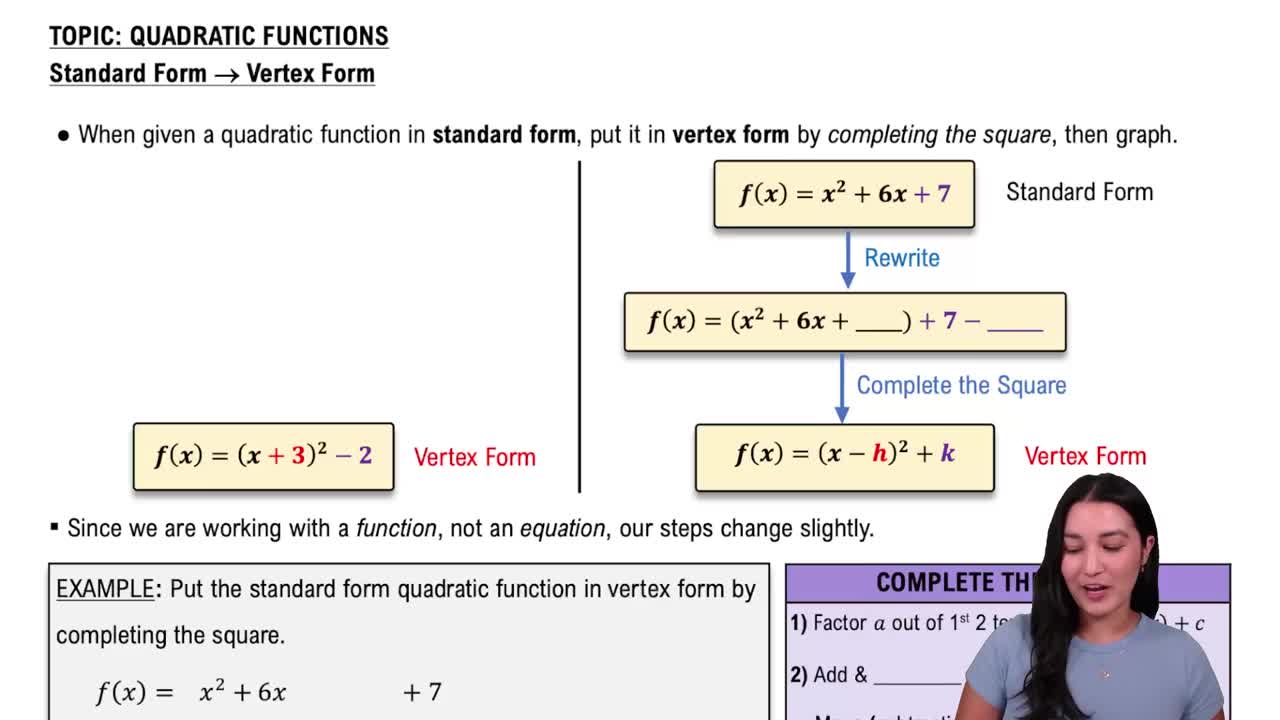
Converting Standard Form to Vertex Form

 5:35m
5:35mWatch next
Master Introduction to Quadratic Equations with a bite sized video explanation from Callie
Start learningRelated Videos
Related Practice