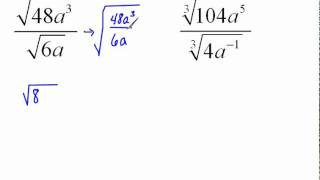In Exercises 73–84, simplify each expression using the quotients-to-powers rule. (- 3x/y)⁴

Verified step by step guidance

1. Identify the expression to simplify: \((-\frac{3x}{y})^4\).
2. Apply the power of a quotient rule: \(\left(\frac{a}{b}\right)^n = \frac{a^n}{b^n}\).
3. Rewrite the expression using the rule: \(\frac{(-3x)^4}{y^4}\).
4. Apply the power of a product rule: \((ab)^n = a^n b^n\).
5. Simplify \((-3x)^4\) to \((-3)^4 \cdot x^4\).

Key Concepts

Quotients-to-Powers Rule

The quotients-to-powers rule states that when raising a fraction to a power, you can apply the exponent to both the numerator and the denominator separately. This means that for any expression of the form (a/b)ⁿ, it can be simplified to aⁿ/bⁿ. This rule is essential for simplifying expressions involving fractions raised to exponents.

Exponent Rules

Exponent rules are a set of mathematical principles that govern how to manipulate powers and roots. Key rules include the product of powers, power of a power, and power of a product. Understanding these rules is crucial for simplifying expressions, especially when dealing with multiple terms raised to exponents, as they dictate how to combine and simplify these terms effectively.

Negative Exponents

Negative exponents indicate the reciprocal of the base raised to the opposite positive exponent. For example, x⁻ⁿ = 1/xⁿ. This concept is important when simplifying expressions, as it allows for the transformation of terms that may appear in the denominator into a more manageable form in the numerator, facilitating easier simplification of the overall expression.