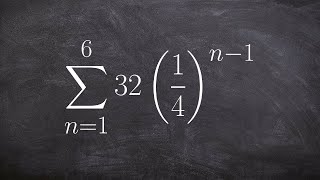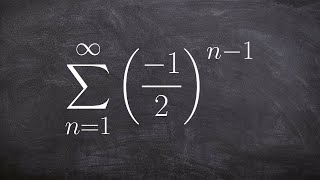Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
9. Sequences, Series, & Induction
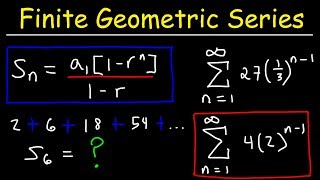
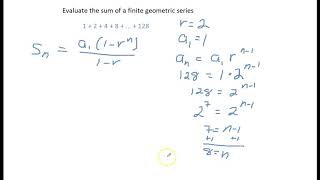
Geometric Sequences
Problem 53a
Textbook Question
In Exercises 51–56, the general term of a sequence is given. Determine whether the sequence is arithmetic, geometric, or neither. If the sequence is arithmetic, find the common difference; if it is geometric, find the common ratio. an = 2^n
 Verified step by step guidance
Verified step by step guidance1
<Start by understanding the general term of the sequence, which is given as a_n = 2^n.>
<To determine if the sequence is arithmetic, check if the difference between consecutive terms is constant. Calculate a_2 - a_1, a_3 - a_2, and so on.>
<To determine if the sequence is geometric, check if the ratio between consecutive terms is constant. Calculate a_2 / a_1, a_3 / a_2, and so on.>
<If the difference is constant, the sequence is arithmetic, and the constant difference is the common difference.>
<If the ratio is constant, the sequence is geometric, and the constant ratio is the common ratio.>
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Arithmetic Sequence
An arithmetic sequence is a sequence of numbers in which the difference between consecutive terms is constant. This difference is known as the common difference. For example, in the sequence 2, 4, 6, 8, the common difference is 2. To determine if a sequence is arithmetic, one can subtract each term from the subsequent term and check for consistency.
Recommended video:
Guided course

Arithmetic Sequences - General Formula
Geometric Sequence
A geometric sequence is a sequence where each term after the first is found by multiplying the previous term by a fixed, non-zero number called the common ratio. For instance, in the sequence 3, 6, 12, 24, the common ratio is 2. To identify a geometric sequence, one can divide each term by its preceding term and verify if the ratio remains constant.
Recommended video:
Guided course

Geometric Sequences - Recursive Formula
Exponential Functions
Exponential functions are mathematical functions of the form f(n) = a * b^n, where 'a' is a constant, 'b' is the base, and 'n' is the exponent. In the given sequence an = 2^n, it represents an exponential growth pattern where each term is a power of 2. Understanding exponential functions is crucial for analyzing sequences that grow or decay at rates proportional to their current value.
Recommended video:

Exponential Functions

 4:18m
4:18mWatch next
Master Geometric Sequences - Recursive Formula with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice