Evaluate each determinant.

Verified step by step guidance

1. Identify the size of the matrix for which you need to evaluate the determinant. For a 2x2 matrix, use the formula: \( \text{det}(A) = ad - bc \) where \( A = \begin{bmatrix} a & b \\ c & d \end{bmatrix} \).
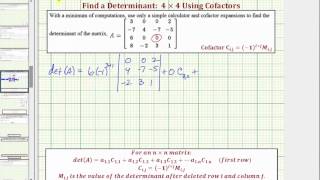
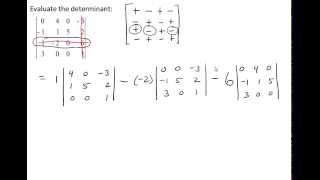
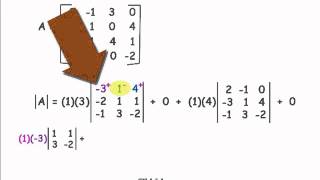
2. For a 3x3 matrix, use the rule of Sarrus or the cofactor expansion method. The rule of Sarrus is applicable only for 3x3 matrices and involves summing the products of the diagonals from left to right and subtracting the products of the diagonals from right to left.
3. If using cofactor expansion for a 3x3 matrix, choose a row or column to expand along. Calculate the determinant by summing the products of each element in the row or column with its corresponding cofactor.
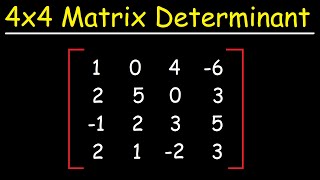
4. For larger matrices (4x4 or more), use the method of cofactor expansion or reduce the matrix to an upper triangular form using row operations, then multiply the diagonal elements to find the determinant.
5. Remember that the determinant can be used to determine if a matrix is invertible (non-zero determinant) or singular (zero determinant).

Key Concepts

Determinant of a Matrix

A determinant is a scalar value that can be computed from the elements of a square matrix. It provides important information about the matrix, such as whether it is invertible (non-zero determinant) or singular (zero determinant). The determinant can be calculated using various methods, including row reduction, cofactor expansion, or specific formulas for small matrices.

Properties of Determinants

Determinants have several key properties that simplify their evaluation. For instance, the determinant of a product of matrices equals the product of their determinants, and swapping two rows of a matrix changes the sign of the determinant. Additionally, if two rows are identical, the determinant is zero, which indicates linear dependence among the rows.

Applications of Determinants

Determinants are used in various applications, including solving systems of linear equations, finding the area or volume of geometric shapes, and analyzing the stability of systems in engineering. They also play a crucial role in calculus, particularly in the context of Jacobians when changing variables in multiple integrals.