Here are the essential concepts you must grasp in order to answer the question correctly.
Perfect Square Trinomial
A perfect square trinomial is a quadratic expression that can be expressed as the square of a binomial. It takes the form (a + b)² = a² + 2ab + b², where 'a' and 'b' are real numbers. Recognizing this structure is essential for transforming a given binomial into a perfect square trinomial by identifying the necessary constant to add.
Recommended video:
Solving Quadratic Equations by Completing the Square
Completing the Square
Completing the square is a method used to convert a quadratic expression into a perfect square trinomial. This involves taking half of the coefficient of the linear term, squaring it, and adding it to the expression. For the binomial x² + 3x, half of 3 is 1.5, and squaring it gives 2.25, which is the constant needed to complete the square.
Recommended video:
Solving Quadratic Equations by Completing the Square
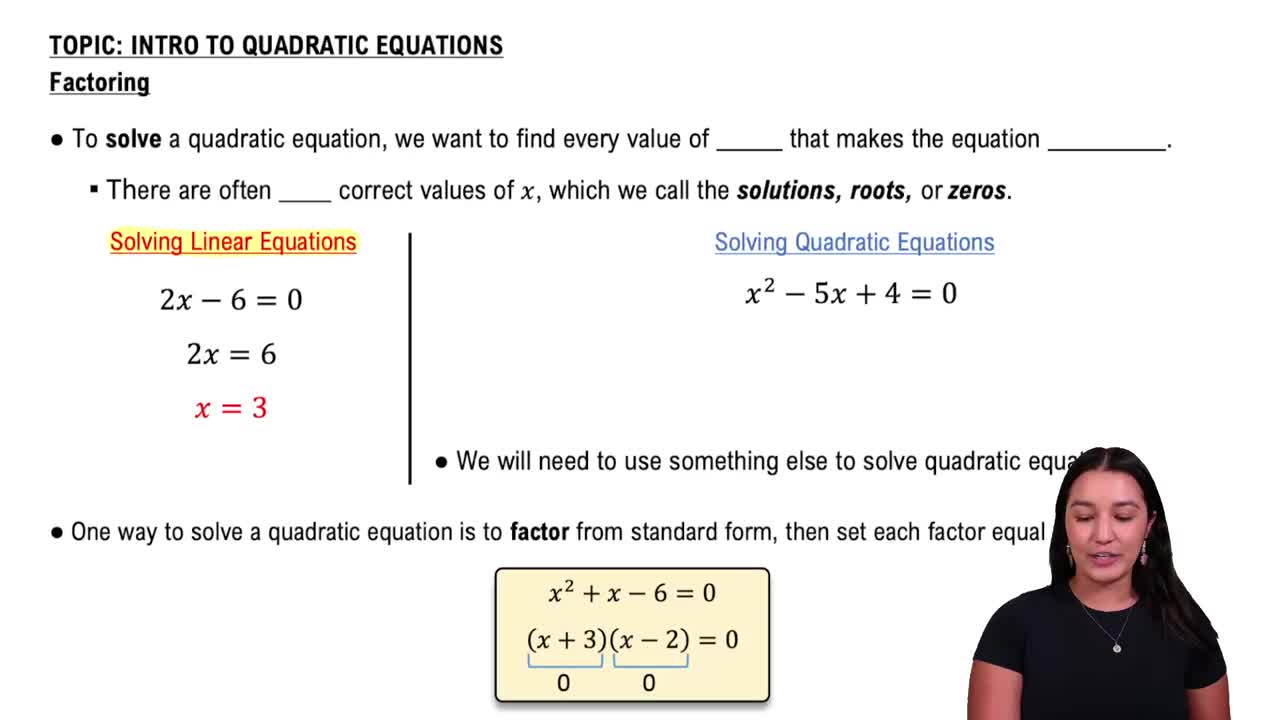
Factoring Quadratics
Factoring quadratics involves rewriting a quadratic expression as a product of its linear factors. Once the expression is transformed into a perfect square trinomial, it can be factored into the form (x + b)². This step is crucial for simplifying expressions and solving equations, as it allows for easier manipulation and understanding of the roots of the quadratic.
Recommended video:
Solving Quadratic Equations by Factoring
 Verified Solution
Verified Solution


 5:35m
5:35m
