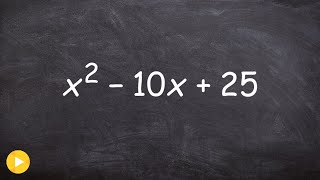Find all values of b or c that will make the polynomial a perfect square trinomial. 49x^2+70x+c

Verified step by step guidance

1. Identify the structure of a perfect square trinomial, which is of the form \((ax + b)^2 = a^2x^2 + 2abx + b^2\).
2. Compare the given polynomial \(49x^2 + 70x + c\) with the perfect square trinomial form \(a^2x^2 + 2abx + b^2\).
3. Notice that \(49x^2\) corresponds to \(a^2x^2\), so \(a^2 = 49\). Solve for \(a\) to find \(a = 7\) or \(a = -7\).
4. The term \(70x\) corresponds to \(2abx\). Substitute \(a = 7\) into \(2ab = 70\) to solve for \(b\).
5. Calculate \(b^2\) to find \(c\) by using the equation \(c = b^2\).

Key Concepts

Perfect Square Trinomial

A perfect square trinomial is a quadratic expression that can be written in the form (ax + b)², which expands to a²x² + 2abx + b². For a trinomial to be a perfect square, the first term must be a perfect square, the last term must also be a perfect square, and the middle term must be twice the product of the square roots of the first and last terms.

Coefficients in Quadratics

In the polynomial 49x² + 70x + c, the coefficients are the numerical factors of each term. The coefficient of x² is 49, which is a perfect square (7²), and the coefficient of x is 70. Understanding how these coefficients relate to the conditions for forming a perfect square trinomial is essential for determining the value of c.

Finding c for Perfect Square

To find the value of c that makes the polynomial a perfect square trinomial, we can use the relationship between the coefficients. Specifically, we set c equal to the square of half the coefficient of x, which is (70/2)² = 35² = 1225. Thus, c must equal 1225 for the polynomial to be a perfect square trinomial.