Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
2. Graphs of Equations
Lines
Problem 71
Textbook Question
Use a graphing calculator to solve each linear equation. 3(2x+1) - 2 (x-2) =5
 Verified step by step guidance
Verified step by step guidance1
Distribute the numbers outside the parentheses: Multiply 3 by each term inside the first parentheses and -2 by each term inside the second parentheses.
Simplify the equation by combining like terms.
Isolate the variable term by moving constant terms to the other side of the equation.
Divide both sides of the equation by the coefficient of the variable to solve for x.
Use a graphing calculator to verify the solution by graphing the equation and checking the x-intercept.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
1mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Linear Equations
A linear equation is an algebraic expression that represents a straight line when graphed. It typically takes the form ax + b = c, where a, b, and c are constants. Understanding how to manipulate and solve these equations is essential for finding the value of the variable, in this case, x.
Recommended video:

Categorizing Linear Equations
Graphing Calculators
Graphing calculators are powerful tools that allow users to visualize equations and perform complex calculations. They can plot graphs of functions, solve equations, and provide numerical solutions. Familiarity with using a graphing calculator is crucial for efficiently solving linear equations and interpreting their graphical representations.
Recommended video:

Transformations of Exponential Graphs
Distributive Property
The distributive property is a fundamental algebraic principle that states a(b + c) = ab + ac. It allows for the multiplication of a single term across terms within parentheses. In the context of the given equation, applying the distributive property is necessary to simplify the expression before solving for x.
Recommended video:
Guided course
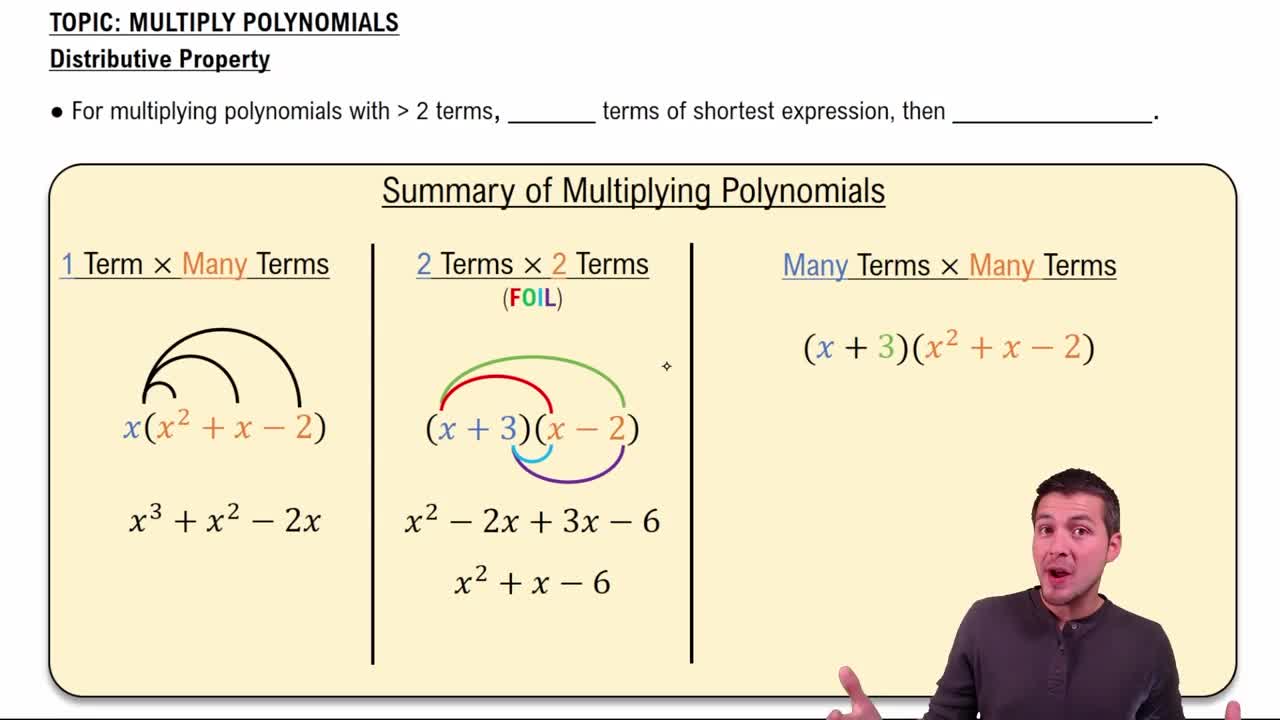
Multiply Polynomials Using the Distributive Property

 6:49m
6:49mWatch next
Master The Slope of a Line with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice