Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
4. Polynomial Functions
Understanding Polynomial Functions
Problem 28a
Textbook Question
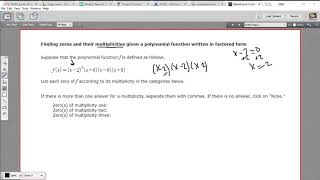
Textbook QuestionIn Exercises 25–32, find the zeros for each polynomial function and give the multiplicity for each zero. State whether the graph crosses the x-axis, or touches the x-axis and turns around, at each zero. f(x)=−3(x+1/2)(x−4)^3
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
3mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Zeros of a Polynomial
The zeros of a polynomial function are the values of x for which the function equals zero. These points are where the graph intersects the x-axis. To find the zeros, we set the polynomial equal to zero and solve for x, which often involves factoring or using the quadratic formula for higher-degree polynomials.
Recommended video:

Finding Zeros & Their Multiplicity
Multiplicity of Zeros
The multiplicity of a zero refers to the number of times a particular zero appears as a factor in the polynomial. If a zero has an odd multiplicity, the graph will cross the x-axis at that zero. Conversely, if a zero has an even multiplicity, the graph will touch the x-axis and turn around at that point.
Recommended video:

Finding Zeros & Their Multiplicity
Graph Behavior at Zeros
The behavior of a polynomial graph at its zeros is determined by the multiplicity of each zero. A zero with odd multiplicity results in the graph crossing the x-axis, while a zero with even multiplicity causes the graph to touch the x-axis and reverse direction. Understanding this behavior helps in sketching the graph and predicting its shape around the zeros.
Recommended video:

Identifying Intervals of Unknown Behavior

 6:04m
6:04mWatch next
Master Introduction to Polynomial Functions with a bite sized video explanation from Callie
Start learningRelated Videos
Related Practice