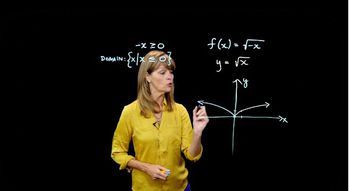In Exercises 64–66, begin by graphing the square root function, f(x) = √x. Then use transformations of this graph to graph the given function. g(x) = √(x + 3)

Verified step by step guidance

1. Start by graphing the basic square root function, \( f(x) = \sqrt{x} \). This graph begins at the origin (0,0) and increases gradually to the right, forming a curve that gets less steep as x increases.
2. Identify the transformation needed to graph \( g(x) = \sqrt{x + 3} \). Notice that the expression inside the square root, \( x + 3 \), indicates a horizontal shift.
3. Understand that adding a constant inside the square root function, \( \sqrt{x + 3} \), results in a horizontal shift to the left by that constant. In this case, the graph of \( f(x) = \sqrt{x} \) will shift 3 units to the left.
4. Apply the transformation to the graph of \( f(x) = \sqrt{x} \). Shift every point on the graph of \( f(x) \) 3 units to the left to obtain the graph of \( g(x) = \sqrt{x + 3} \).
5. Verify the transformation by checking key points. For example, the point (0,0) on \( f(x) \) will move to (-3,0) on \( g(x) \), and the point (1,1) will move to (-2,1).

Key Concepts

Square Root Function

The square root function, f(x) = √x, is defined for x ≥ 0 and produces non-negative outputs. Its graph is a curve that starts at the origin (0,0) and increases gradually, reflecting the relationship between x and its square root. Understanding this function is crucial as it serves as the foundation for applying transformations.

Graph Transformations

Graph transformations involve shifting, stretching, compressing, or reflecting the graph of a function. In this case, the transformation g(x) = √(x + 3) represents a horizontal shift of the square root function to the left by 3 units. Recognizing how these transformations affect the original graph is essential for accurately graphing the new function.

Horizontal Shifts

Horizontal shifts occur when a function is modified by adding or subtracting a constant inside the function's argument. For g(x) = √(x + 3), the '+3' indicates a shift to the left, meaning every point on the graph of f(x) = √x moves left by 3 units. This concept is vital for understanding how the graph of g(x) relates to f(x).