Here are the essential concepts you must grasp in order to answer the question correctly.
Rational Expressions
Rational expressions are fractions where the numerator and denominator are polynomials. Understanding how to manipulate these expressions is crucial for solving equations involving them. In this case, the equation contains rational expressions that require common denominators for simplification and solving.
Recommended video:
Rationalizing Denominators
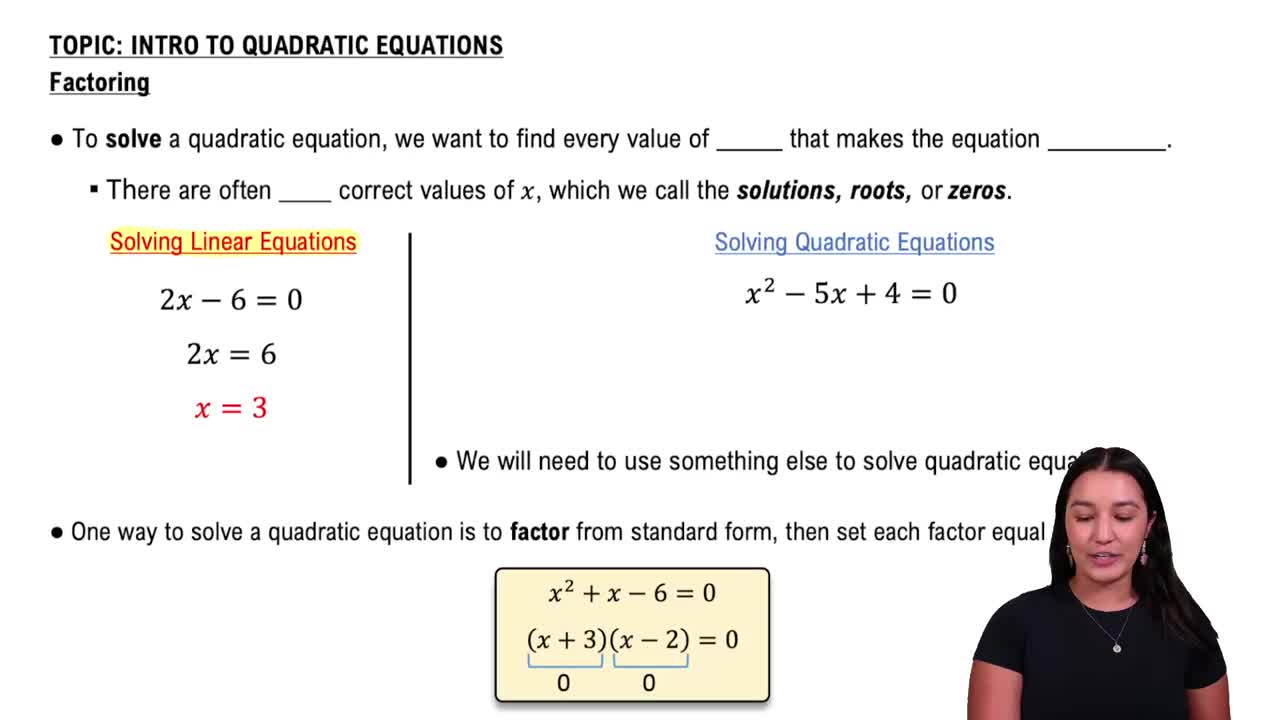
Solving Quadratic Equations
Quadratic equations are polynomial equations of degree two, typically in the form ax^2 + bx + c = 0. To solve them, one can use methods such as factoring, completing the square, or the quadratic formula. In the given equation, after simplification, one may end up with a quadratic that needs to be solved.
Recommended video:
Solving Quadratic Equations by Factoring
Finding Common Denominators
Finding a common denominator is essential when adding or subtracting rational expressions. It allows for the combination of fractions into a single expression. In the provided equation, both sides contain terms with the denominator (x-1), which must be addressed to simplify and solve the equation effectively.
Recommended video:
Rationalizing Denominators
 Verified Solution
Verified Solution


 5:56m
5:56m
