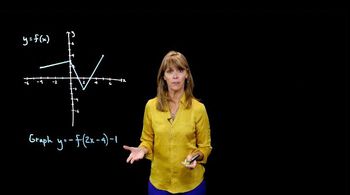In Exercises 17-32, use the graph of y = f(x) to graph each function g.
g(x) = ½ f(x)

Verified step by step guidance

1. Identify the given function: The graph provided is of the function y = f(x).
2. Understand the transformation: The function g(x) = \frac{1}{2}f(x) represents a vertical compression of the graph of f(x) by a factor of \frac{1}{2}.
3. Apply the transformation to key points: For each point (x, y) on the graph of f(x), the corresponding point on the graph of g(x) will be (x, \frac{1}{2}y).
4. Transform the given points: For the point (3, 0) on f(x), the corresponding point on g(x) is (3, \frac{1}{2} \times 0) = (3, 0). For the point (0, -27) on f(x), the corresponding point on g(x) is (0, \frac{1}{2} \times -27) = (0, -13.5).
5. Sketch the graph of g(x): Plot the transformed points and draw the graph of g(x) = \frac{1}{2}f(x) using the same shape as f(x) but vertically compressed.

Key Concepts

Function Transformation

Function transformation refers to the process of altering the graph of a function through various operations, such as vertical or horizontal shifts, stretches, or reflections. In this case, the function g(x) = ½ f(x) represents a vertical compression of the original function f(x) by a factor of ½, meaning that all y-values of f(x) are halved.

Graphing Functions

Graphing functions involves plotting points on a coordinate plane to visually represent the relationship between the input (x) and output (y) values of a function. Understanding how to read and interpret the graph of f(x) is essential for accurately graphing g(x), as it allows one to apply the transformation rules effectively.

Key Points on a Graph

Key points on a graph, such as intercepts and turning points, are critical for understanding the behavior of a function. In the provided graph, the points (3, 0) and (0, -27) are significant as they indicate where the function intersects the axes. These points will also be transformed when graphing g(x), helping to maintain the overall shape of the function while applying the vertical compression.