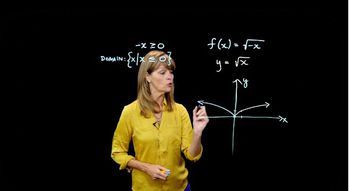Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
3. Functions
Transformations
Problem 33b
Textbook Question
Graph each function. See Examples 1 and 2. ƒ(x)=-√-x
 Verified step by step guidance
Verified step by step guidance1
Step 1: Understand the function. The function given is ƒ(x)=-√-x. This is a square root function, which is reflected over the x-axis (due to the negative sign) and over the y-axis (due to the negative sign inside the square root).
Step 2: Identify the domain of the function. The domain of a square root function is all x-values for which the expression inside the square root is greater than or equal to zero. In this case, the expression inside the square root is -x, so the domain is all x-values for which -x ≥ 0. Solving this inequality gives x ≤ 0.
Step 3: Identify the range of the function. The range of a square root function is all y-values for which the function is defined. Since the function is reflected over the x-axis, the range is all y-values less than or equal to zero.
Step 4: Plot the function. Start by plotting the point (0,0), which is the vertex of the graph. Then, for x-values less than zero, plot points that are the negative square root of the absolute value of x. For example, you could plot the points (-1,-1), (-4,-2), and (-9,-3).
Step 5: Draw the graph. Connect the points with a smooth curve to complete the graph of the function. Remember that the graph should only exist for x-values less than or equal to zero and y-values less than or equal to zero, due to the domain and range of the function.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
5mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Graphing Functions
Graphing functions involves plotting points on a coordinate plane to visualize the relationship between the input (x) and output (f(x)). Understanding how to interpret the function's equation is crucial, as it dictates the shape and position of the graph. For example, the function f(x) = -√-x will have specific characteristics based on its domain and range, which must be considered when graphing.
Recommended video:

Graphs of Logarithmic Functions
Domain and Range
The domain of a function refers to all possible input values (x) that can be used without causing any mathematical errors, such as taking the square root of a negative number. For the function f(x) = -√-x, the domain is limited to non-positive values of x. The range, on the other hand, represents all possible output values (f(x)), which in this case will be non-positive as well, since the square root function outputs non-negative values that are then negated.
Recommended video:

Domain & Range of Transformed Functions
Transformations of Functions
Transformations of functions involve shifting, reflecting, stretching, or compressing the graph of a function. In the case of f(x) = -√-x, the negative sign in front of the square root indicates a reflection across the x-axis, while the negative inside the square root indicates a horizontal reflection. Understanding these transformations helps in accurately sketching the graph based on the original function's characteristics.
Recommended video:

Domain & Range of Transformed Functions

 5:25m
5:25mWatch next
Master Intro to Transformations with a bite sized video explanation from Nick Kaneko
Start learningRelated Videos
Related Practice