Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
5. Rational Functions
Graphing Rational Functions
Problem 86
Textbook Question
Graph each rational function. See Examples 5–9. ƒ(x)=(18+6x-4x^2)/(4+6x+2x^2)
 Verified step by step guidance
Verified step by step guidance1
Identify the rational function: \( f(x) = \frac{18 + 6x - 4x^2}{4 + 6x + 2x^2} \).
Factor both the numerator and the denominator, if possible, to simplify the function.
Determine the vertical asymptotes by setting the denominator equal to zero and solving for \( x \).
Find the horizontal asymptote by comparing the degrees of the numerator and the denominator.
Plot the intercepts by setting \( x = 0 \) to find the y-intercept and setting \( f(x) = 0 \) to find the x-intercepts.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
9mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Rational Functions
A rational function is a function that can be expressed as the ratio of two polynomials. The general form is f(x) = P(x)/Q(x), where P(x) and Q(x) are polynomials. Understanding the behavior of rational functions involves analyzing their asymptotes, intercepts, and overall shape, which are influenced by the degrees and coefficients of the polynomials.
Recommended video:

Intro to Rational Functions
Asymptotes
Asymptotes are lines that a graph approaches but never touches. For rational functions, vertical asymptotes occur where the denominator equals zero, indicating points of discontinuity. Horizontal asymptotes describe the behavior of the function as x approaches infinity, determined by the degrees of the numerator and denominator. Identifying these asymptotes is crucial for accurately graphing the function.
Recommended video:

Introduction to Asymptotes
Intercepts
Intercepts are points where the graph of a function crosses the axes. The x-intercepts occur when f(x) = 0, which means the numerator of the rational function must equal zero. The y-intercept is found by evaluating f(0). Identifying these intercepts helps in sketching the graph and understanding the function's behavior at specific points.
Recommended video:
Guided course

Graphing Intercepts

 5:31m
5:31mWatch next
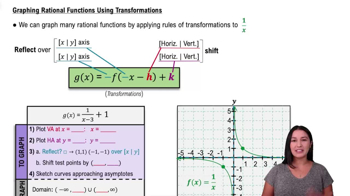
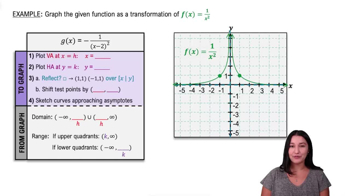
Master Graphing Rational Functions Using Transformations with a bite sized video explanation from Callie
Start learningRelated Videos
Related Practice