Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
7. Systems of Equations & Matrices
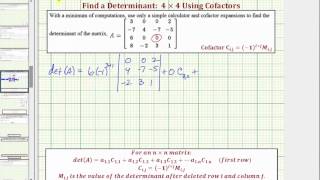
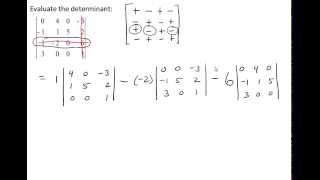
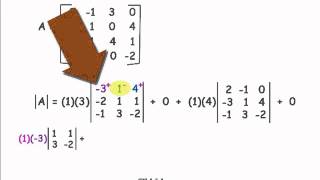
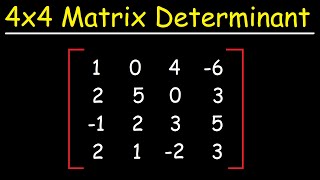
Determinants and Cramer's Rule
Problem 9d
Textbook Question
Are the given matrices inverses of each other? (Hint: Check to see whether their products are the identity matrix I↓n.) [2x2 matrix] and [2x2 matrix]
 Verified step by step guidance
Verified step by step guidance1
Step 1: Understand the concept of inverse matrices. Two matrices A and B are inverses of each other if their product is the identity matrix. For 2x2 matrices, the identity matrix is I = \begin{bmatrix} 1 & 0 \\ 0 & 1 \end{bmatrix}.
Step 2: Multiply the given matrices. Let's denote the first matrix as A and the second matrix as B. Calculate the product AB by performing matrix multiplication.
Step 3: Perform the matrix multiplication. For matrices A = \begin{bmatrix} a & b \\ c & d \end{bmatrix} and B = \begin{bmatrix} e & f \\ g & h \end{bmatrix}, the product AB is \begin{bmatrix} ae + bg & af + bh \\ ce + dg & cf + dh \end{bmatrix}.
Step 4: Check if the resulting matrix from the multiplication is the identity matrix I = \begin{bmatrix} 1 & 0 \\ 0 & 1 \end{bmatrix}.
Step 5: If the product AB equals the identity matrix, then the matrices are inverses of each other. If not, they are not inverses.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
3mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Matrix Multiplication
Matrix multiplication involves combining two matrices to produce a third matrix. For two matrices A and B to be multiplied, the number of columns in A must equal the number of rows in B. The resulting matrix's elements are calculated by taking the dot product of the rows of A with the columns of B. Understanding this operation is crucial for determining if two matrices are inverses.
Recommended video:

Finding Zeros & Their Multiplicity
Identity Matrix
An identity matrix is a square matrix with ones on the diagonal and zeros elsewhere. For a 2x2 matrix, the identity matrix is represented as I = [[1, 0], [0, 1]]. When a matrix A is multiplied by its inverse A⁻¹, the result is the identity matrix. This property is essential for verifying if two matrices are inverses of each other.
Recommended video:
Guided course

Introduction to Matrices
Inverse of a Matrix
The inverse of a matrix A, denoted A⁻¹, is a matrix that, when multiplied by A, yields the identity matrix. Not all matrices have inverses; a matrix must be square and have a non-zero determinant to possess an inverse. Checking if the product of two matrices equals the identity matrix is the primary method for confirming their inverse relationship.
Recommended video:

Graphing Logarithmic Functions

 4:36m
4:36mWatch next
Master Determinants of 2×2 Matrices with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice