Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
0. Review of Algebra
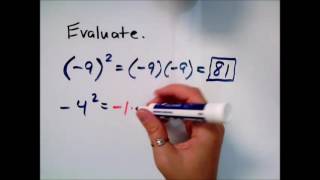
Exponents
Problem 147
Textbook Question
Write each fraction as a decimal. For repeating decimals, write the answer by first using bar notation and then rounding to the nearest thousandth. 5/9
 Verified step by step guidance
Verified step by step guidance1
Step 1: Understand that to convert a fraction to a decimal, you need to perform division. Here, divide 5 by 9.
Step 2: Set up the division of 5 by 9. Since 5 is less than 9, you will need to add a decimal point and zeros to 5 to perform the division.
Step 3: Perform the long division. Notice that after a few steps, the remainder starts repeating, indicating a repeating decimal.
Step 4: Identify the repeating part of the decimal. Use bar notation to represent the repeating sequence.
Step 5: Round the repeating decimal to the nearest thousandth by considering the digits up to the fourth decimal place.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Fractions and Decimals
Fractions represent a part of a whole and can be converted into decimals. The numerator indicates how many parts are taken, while the denominator shows the total number of equal parts. Understanding this relationship is crucial for converting fractions like 5/9 into their decimal form.
Recommended video:
Guided course

Radical Expressions with Fractions
Repeating Decimals
Repeating decimals occur when a decimal representation of a fraction has one or more digits that repeat indefinitely. For example, 5/9 converts to 0.555..., where '5' repeats. Bar notation is used to denote this repetition, such as 0.5̅, indicating that the '5' continues infinitely.
Recommended video:

How to Graph Rational Functions
Rounding Decimals
Rounding decimals involves adjusting the decimal to a specified number of places, which simplifies the number while maintaining its value. When rounding to the nearest thousandth, you look at the digit in the fourth decimal place to determine whether to round up or down. This is essential for providing a concise answer when dealing with repeating decimals.
Recommended video:

The Number e

 7:39m
7:39mWatch next
Master Introduction to Exponent Rules with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice