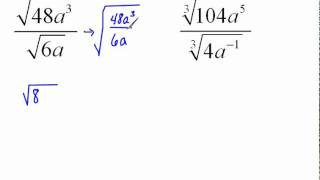Simplify each expression. Write answers without negative exponents. Assume all vari-ables represent positive real numbers. See Examples 8 and 9. 8^2/3

Verified step by step guidance

1. Identify the expression: $8^{2/3}$.
2. Recognize that the expression involves a fractional exponent, which can be rewritten using radicals.
3. Rewrite $8^{2/3}$ as $(8^{1/3})^2$.
4. Understand that $8^{1/3}$ represents the cube root of 8.
5. Calculate the cube root of 8, then square the result to simplify the expression.

Key Concepts

Exponents and Powers

Exponents represent repeated multiplication of a base number. For example, in the expression 8^2/3, the exponent 2/3 indicates that we first take the cube root of 8 and then square the result. Understanding how to manipulate exponents is crucial for simplifying expressions involving powers.

Negative Exponents

Negative exponents indicate the reciprocal of the base raised to the absolute value of the exponent. For instance, a term like x^-n can be rewritten as 1/x^n. In the context of this question, simplifying expressions without negative exponents means ensuring all results are expressed in a positive exponent form.

Radical Expressions

Radical expressions involve roots, such as square roots or cube roots. The expression 8^(2/3) can be interpreted as the cube root of 8 squared. Understanding how to convert between radical and exponent forms is essential for simplifying expressions and ensuring clarity in mathematical communication.