Here are the essential concepts you must grasp in order to answer the question correctly.
Quadratic Formula
The quadratic formula is a method for solving quadratic equations of the form ax^2 + bx + c = 0. It is expressed as x = (-b ± √(b² - 4ac)) / (2a), where a, b, and c are coefficients from the equation. This formula provides the solutions for x, which can be real or complex numbers depending on the value of the discriminant (b² - 4ac).
Recommended video:
Solving Quadratic Equations Using The Quadratic Formula
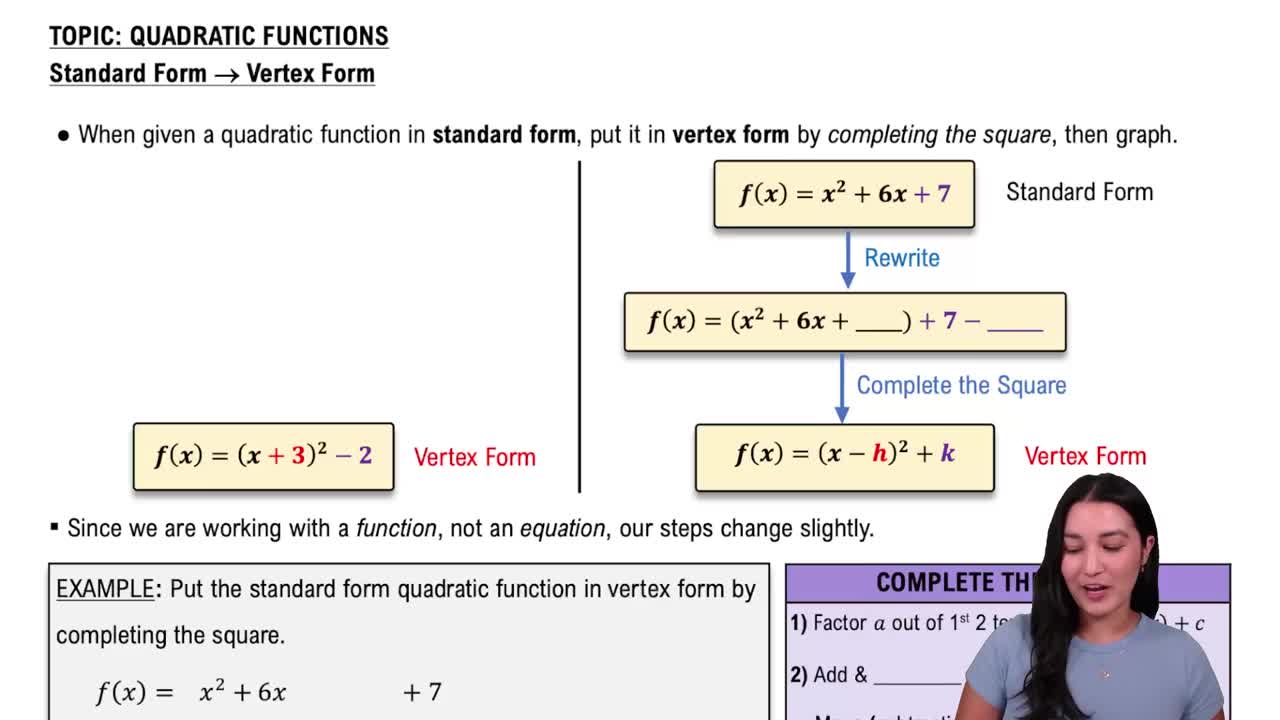
Standard Form of a Quadratic Equation
A quadratic equation is typically written in standard form as ax^2 + bx + c = 0, where a, b, and c are constants, and a ≠ 0. This form is essential for identifying the coefficients needed to apply the quadratic formula. In the given equation, 1/2x^2 + 1/4x - 3 = 0, the coefficients can be extracted to facilitate solving.
Recommended video:
Converting Standard Form to Vertex Form
Discriminant
The discriminant is the part of the quadratic formula under the square root, calculated as b² - 4ac. It determines the nature of the roots of the quadratic equation: if the discriminant is positive, there are two distinct real roots; if it is zero, there is one real root (a repeated root); and if it is negative, there are two complex roots. Understanding the discriminant helps predict the type of solutions before calculating them.
Recommended video:
 Verified step by step guidance
Verified step by step guidance Verified Solution
Verified Solution


 6:12m
6:12m