Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
0. Review of Algebra
Polynomials Intro
Problem 86
Textbook Question
In Exercises 83–90, perform the indicated operation or operations. (3x+5)(2x−9)−(7x−2)(x−1)
 Verified step by step guidance
Verified step by step guidance1
First, apply the distributive property to expand the expression \((3x+5)(2x−9)\). Multiply each term in the first binomial by each term in the second binomial.
Calculate \(3x \cdot 2x\), \(3x \cdot (-9)\), \(5 \cdot 2x\), and \(5 \cdot (-9)\) to expand the first part.
Next, apply the distributive property to expand the expression \((7x−2)(x−1)\). Multiply each term in the first binomial by each term in the second binomial.
Calculate \(7x \cdot x\), \(7x \cdot (-1)\), \(-2 \cdot x\), and \(-2 \cdot (-1)\) to expand the second part.
Combine like terms from both expanded expressions and simplify the expression by subtracting the second expanded expression from the first.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
4mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Polynomial Multiplication
Polynomial multiplication involves distributing each term in one polynomial to every term in another polynomial. This process, often referred to as the FOIL method for binomials, ensures that all combinations of terms are accounted for. For example, in the expression (3x + 5)(2x - 9), you would multiply 3x by 2x, 3x by -9, 5 by 2x, and 5 by -9, combining like terms afterward.
Recommended video:

Finding Zeros & Their Multiplicity
Combining Like Terms
Combining like terms is a fundamental algebraic process that simplifies expressions by merging terms that have the same variable raised to the same power. For instance, in the expression 6x^2 + 3x - 2x^2 + 4, the like terms 6x^2 and -2x^2 can be combined to yield 4x^2. This step is crucial for simplifying the result of polynomial operations.
Recommended video:

Combinations
Distributive Property
The distributive property states that a(b + c) = ab + ac, allowing for the multiplication of a single term by a sum or difference. This property is essential when expanding expressions, such as when subtracting one polynomial from another. In the given problem, applying the distributive property helps to correctly handle the subtraction of the second polynomial from the first, ensuring accurate results.
Recommended video:
Guided course
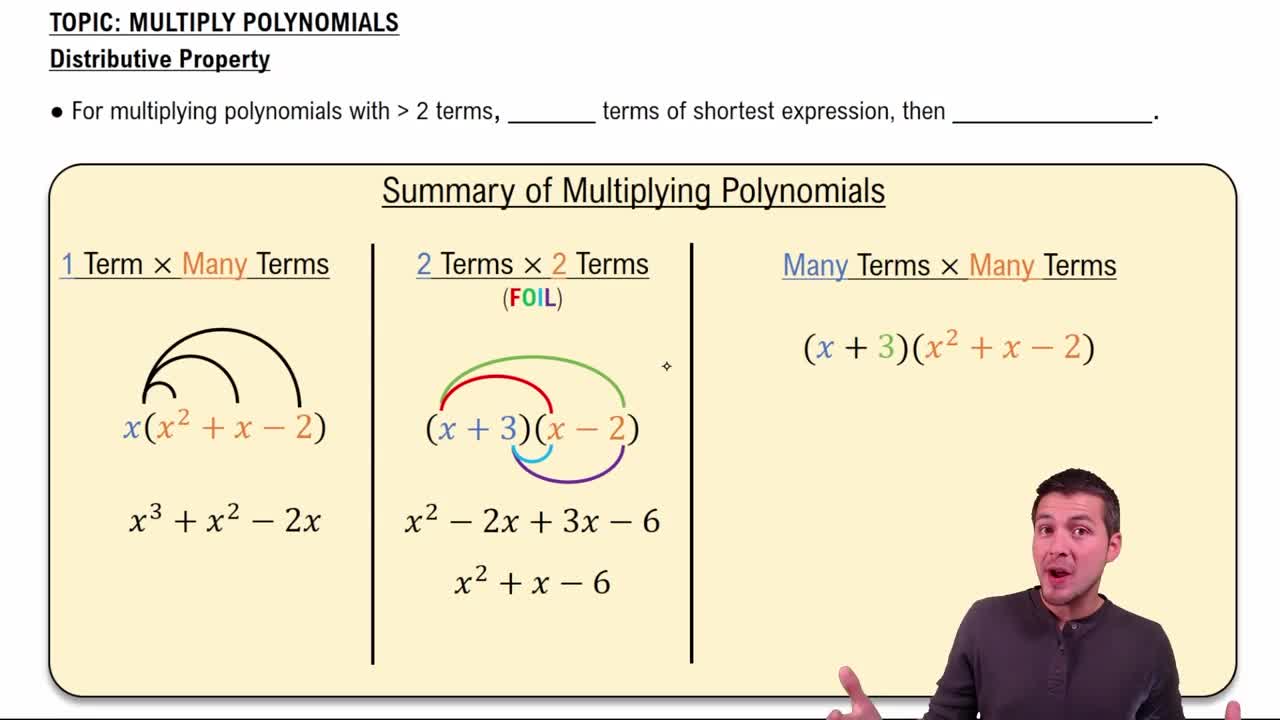
Multiply Polynomials Using the Distributive Property

 5:13m
5:13mWatch next
Master Introduction to Polynomials with a bite sized video explanation from Patrick Ford
Start learning



