Here are the essential concepts you must grasp in order to answer the question correctly.
Imaginary Numbers
Imaginary numbers are defined as multiples of the imaginary unit 'i', where i is the square root of -1. In this problem, the square roots of negative numbers, such as √-6 and √-2, can be expressed as i√6 and i√2, respectively. Understanding how to manipulate imaginary numbers is crucial for simplifying expressions involving square roots of negative values.
Recommended video:
Square Roots of Negative Numbers
Properties of Square Roots
The properties of square roots state that √a * √b = √(a*b) and √a / √b = √(a/b), provided a and b are non-negative. When dealing with negative numbers, these properties still apply, but we must incorporate the imaginary unit. This concept is essential for simplifying the products and quotients of square roots in the given expression.
Recommended video:
Imaginary Roots with the Square Root Property
Simplification of Complex Expressions
Simplifying complex expressions involves combining like terms, reducing fractions, and applying algebraic rules. In this case, after substituting the imaginary components and applying the properties of square roots, one must simplify the resulting expression to its simplest form. This process is vital for arriving at a clear and concise answer.
Recommended video:
 Verified step by step guidance
Verified step by step guidance Verified Solution
Verified Solution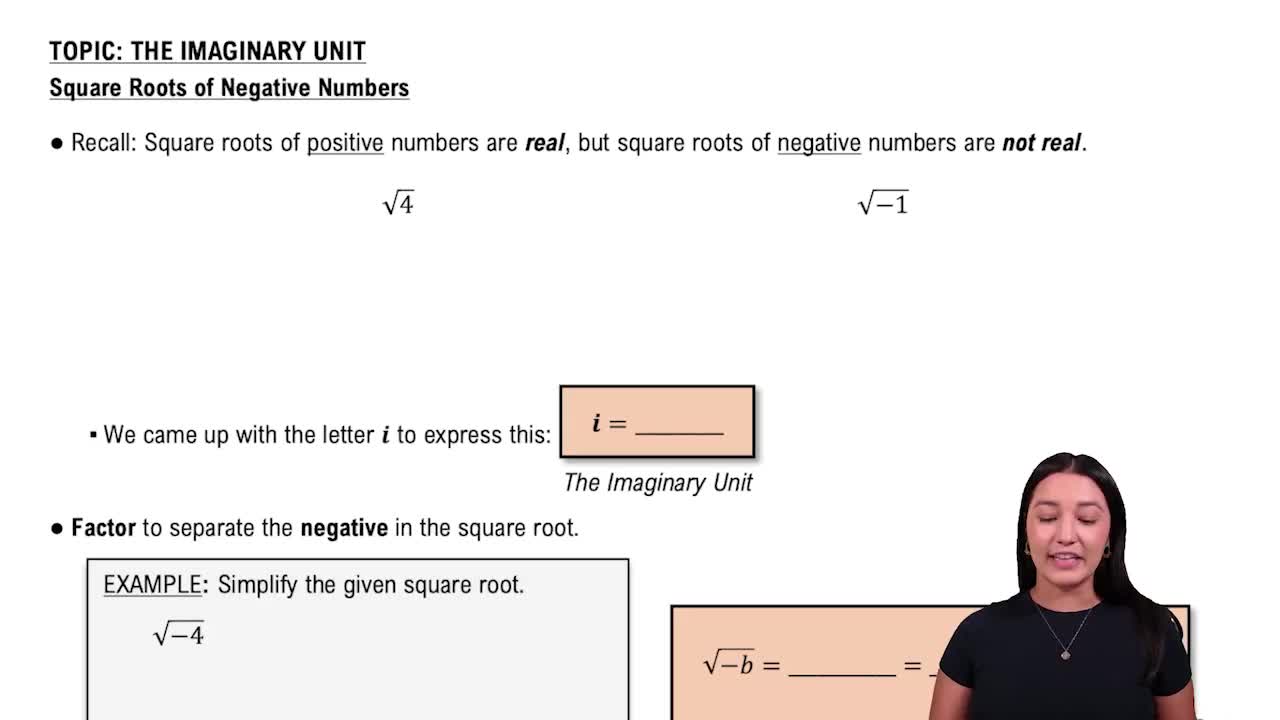



 5:02m
5:02m