Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
7. Systems of Equations & Matrices
Introduction to Matrices
Problem 16a
Textbook Question
In Exercises 14–27, perform the indicated matrix operations given that and D are defined as follows. If an operation is not defined, state the reason. D-A
 Verified step by step guidance
Verified step by step guidance1
Identify the matrices D and A. Ensure you have the correct dimensions for each matrix.
Check if the matrices D and A have the same dimensions. Matrix subtraction is only possible if both matrices have the same number of rows and columns.
If the dimensions match, proceed to subtract matrix A from matrix D. This involves subtracting each element of matrix A from the corresponding element of matrix D.
Write out the resulting matrix after performing the subtraction for each corresponding element.
If the dimensions do not match, state that the operation is not defined because matrix subtraction requires matrices of the same dimensions.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
1mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Matrix Operations
Matrix operations include addition, subtraction, and multiplication of matrices. For addition and subtraction, matrices must have the same dimensions, meaning they must have the same number of rows and columns. Understanding these operations is crucial for performing calculations involving matrices, as they dictate how matrices can be combined or manipulated.
Recommended video:
Guided course

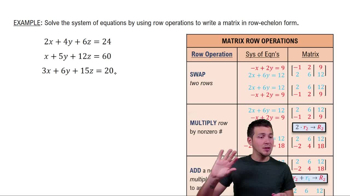
Performing Row Operations on Matrices
Matrix Dimensions
The dimensions of a matrix are defined by the number of rows and columns it contains, expressed as 'm x n' where 'm' is the number of rows and 'n' is the number of columns. When performing operations like addition or subtraction, it is essential to ensure that the matrices involved have the same dimensions; otherwise, the operation is undefined.
Recommended video:
Guided course

Introduction to Matrices
Defined Operations
An operation between matrices is defined only when the matrices involved meet specific criteria, such as having compatible dimensions. For instance, subtracting two matrices is only possible if both matrices have the same dimensions. If the criteria are not met, the operation cannot be performed, and it is important to state the reason for this limitation.
Recommended video:
Guided course

Performing Row Operations on Matrices

 4:35m
4:35mWatch next
Master Introduction to Matrices with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice