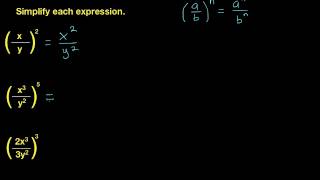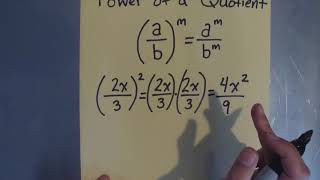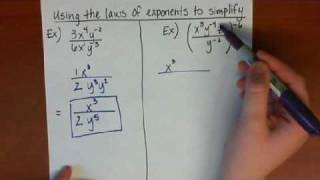In Exercises 1–14, write each number in decimal notation without the use of exponents. 3.8X10²

Verified step by step guidance

1. Identify the given number in scientific notation: \(3.8 \times 10^2\).
2. Understand that \(10^2\) means moving the decimal point 2 places to the right.
3. Start with the number 3.8 and move the decimal point 2 places to the right.
4. After moving the decimal point, fill in any empty spaces with zeros if necessary.
5. Write the final number in decimal notation without exponents.

Key Concepts

Scientific Notation

Scientific notation is a way of expressing numbers that are too large or too small to be conveniently written in decimal form. It is typically formatted as a product of a number between 1 and 10 and a power of ten. For example, 3.8 x 10² means 3.8 multiplied by 100, which simplifies to 380.

Decimal Notation

Decimal notation is the standard way of writing numbers using digits from 0 to 9, where the position of each digit represents a power of ten. Converting from scientific notation to decimal notation involves calculating the value of the exponent and adjusting the decimal point accordingly. This process allows for a clearer understanding of the magnitude of the number.

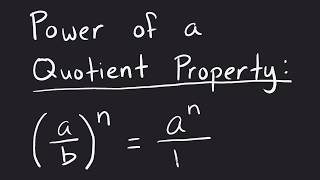
Exponent Rules

Exponent rules are mathematical guidelines that dictate how to handle numbers raised to a power. In the context of scientific notation, the exponent indicates how many places to move the decimal point. A positive exponent means moving the decimal to the right, while a negative exponent would mean moving it to the left, which is essential for converting to decimal notation.