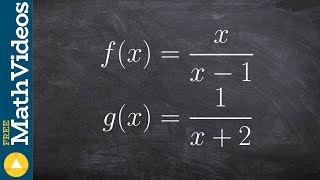In Exercises 82–84, find f + g, f - g, fg, and f/g. f(x) = x^2 + x + 1, g(x) = x^2 -1

Verified step by step guidance

1. To find \( f + g \), add the functions: \( f(x) + g(x) = (x^2 + x + 1) + (x^2 - 1) \). Combine like terms.
2. To find \( f - g \), subtract the functions: \( f(x) - g(x) = (x^2 + x + 1) - (x^2 - 1) \). Distribute the negative sign and combine like terms.
3. To find \( fg \), multiply the functions: \( f(x) \cdot g(x) = (x^2 + x + 1)(x^2 - 1) \). Use the distributive property (FOIL method) to expand the expression.
4. To find \( \frac{f}{g} \), divide the functions: \( \frac{f(x)}{g(x)} = \frac{x^2 + x + 1}{x^2 - 1} \). Simplify the expression if possible, and note any restrictions on the domain where \( g(x) = 0 \).
5. Check for any common factors in the expressions obtained from \( f + g \), \( f - g \), \( fg \), and \( \frac{f}{g} \) to simplify further if possible.

Key Concepts

Function Addition and Subtraction

Function addition and subtraction involve combining two functions by adding or subtracting their outputs for the same input value. For example, if f(x) = x^2 + x + 1 and g(x) = x^2 - 1, then (f + g)(x) = f(x) + g(x) and (f - g)(x) = f(x) - g(x). This process requires careful algebraic manipulation to combine like terms.

Function Multiplication

Function multiplication entails multiplying the outputs of two functions for the same input. For the functions f(x) and g(x), the product is defined as (fg)(x) = f(x) * g(x). This operation often results in a polynomial that can be simplified by distributing the terms and combining like terms, which is essential for further analysis.

Function Division

Function division involves dividing the output of one function by another for the same input. For f(x) and g(x), the quotient is expressed as (f/g)(x) = f(x) / g(x). It is important to note that division by zero is undefined, so one must ensure that g(x) does not equal zero for the values of x being considered.