Here are the essential concepts you must grasp in order to answer the question correctly.
Factoring Quadratic Equations
Factoring quadratic equations involves rewriting the equation in the form of a product of two binomials. This process helps to find the roots of the equation by setting each factor equal to zero. For example, the equation ax^2 + bx + c can often be expressed as (px + q)(rx + s) = 0, where p, q, r, and s are constants.
Recommended video:
Solving Quadratic Equations by Factoring
Zero Product Property
The Zero Product Property states that if the product of two factors equals zero, then at least one of the factors must be zero. This principle is crucial when solving factored equations, as it allows us to set each factor to zero and solve for the variable. For instance, if (x - 2)(x + 3) = 0, then either x - 2 = 0 or x + 3 = 0.
Recommended video:
Product, Quotient, and Power Rules of Logs
Quadratic Formula
The Quadratic Formula provides a method for finding the roots of any quadratic equation in the standard form ax^2 + bx + c = 0. The formula is x = (-b ± √(b² - 4ac)) / (2a). While factoring is often preferred for simpler equations, the quadratic formula is a reliable alternative when factoring is difficult or impossible.
Recommended video:
Solving Quadratic Equations Using The Quadratic Formula
 Verified step by step guidance
Verified step by step guidance Verified Solution
Verified Solution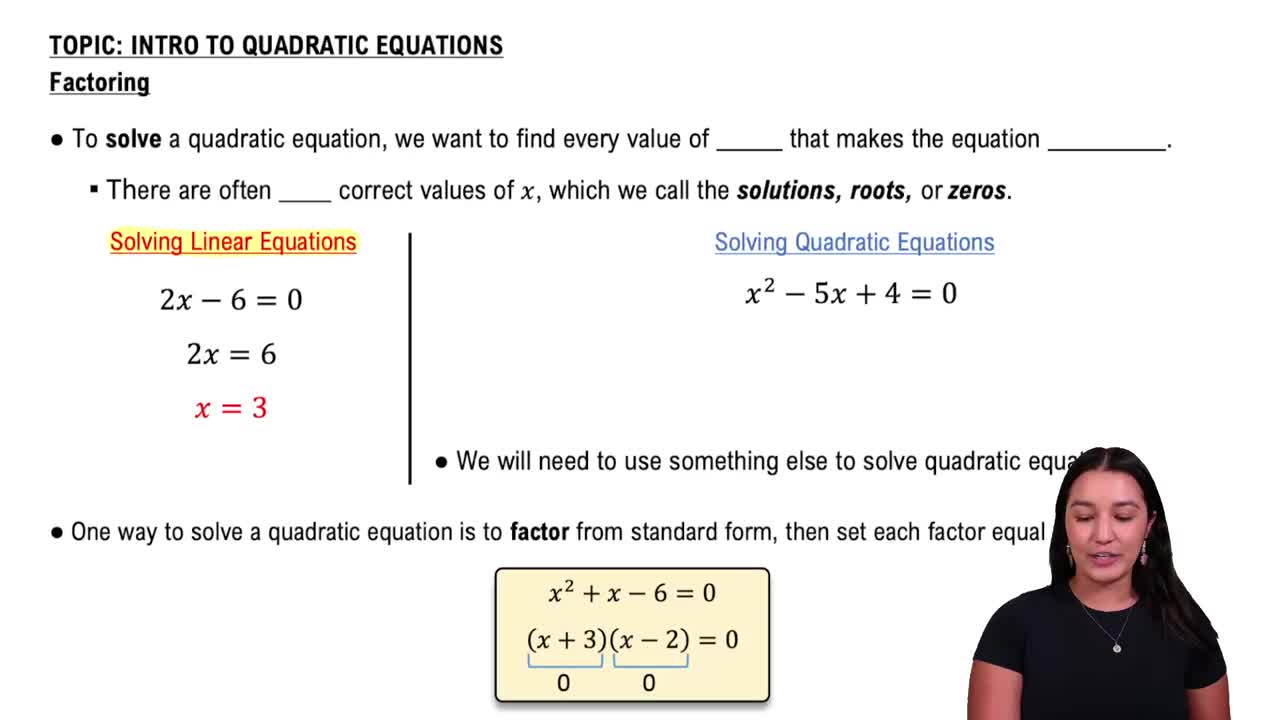



 5:35m
5:35m