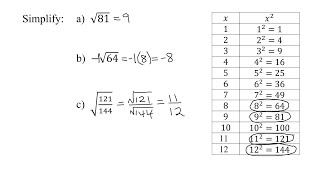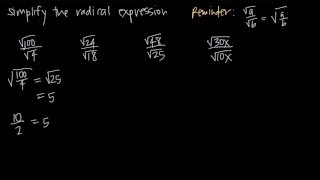Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
0. Review of Algebra
Radical Expressions
Problem 41b
Textbook Question
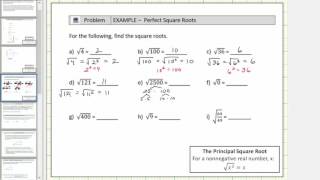
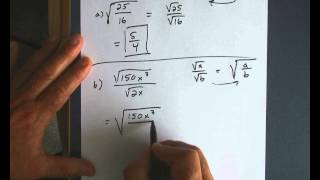
In Exercises 33–46, simplify each expression. _____ −√100x⁶
 Verified step by step guidance
Verified step by step guidance1
Identify the expression under the square root: \( \sqrt{100x^6} \).
Recognize that \( 100x^6 \) can be rewritten as \( (10x^3)^2 \) because \( 10^2 = 100 \) and \( (x^3)^2 = x^6 \).
Apply the property of square roots: \( \sqrt{a^2} = |a| \), which means \( \sqrt{(10x^3)^2} = |10x^3| \).
Since the original expression is \( -\sqrt{100x^6} \), apply the negative sign to the result: \( -|10x^3| \).
Consider the absolute value: \( |10x^3| = 10|x^3| \), so the expression simplifies to \( -10|x^3| \).
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Square Roots
A square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 100 is 10, since 10 × 10 = 100. In algebra, understanding how to simplify square roots, especially with variables, is crucial for solving expressions effectively.
Recommended video:

Imaginary Roots with the Square Root Property
Properties of Exponents
Exponents represent repeated multiplication of a base number. The property of exponents states that x^a * x^b = x^(a+b) and x^a / x^b = x^(a-b). When simplifying expressions involving variables raised to powers, it is essential to apply these properties correctly to combine or reduce terms.
Recommended video:
Guided course

Rational Exponents
Simplifying Radical Expressions
Simplifying radical expressions involves reducing the expression to its simplest form, which often includes factoring out perfect squares from under the radical. For instance, √(x^6) simplifies to x^3, as x^3 * x^3 = x^6. This process is vital for making expressions easier to work with in algebra.
Recommended video:
Guided course

Radical Expressions with Fractions
Related Videos
Related Practice