In Exercises 9–16, use the formula for nCr to evaluate each expression. 7C7

Key Concepts

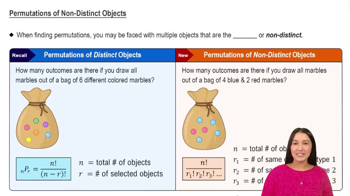
Combination

A combination is a selection of items from a larger set where the order of selection does not matter. In combinatorial mathematics, combinations are denoted as nCr, which represents the number of ways to choose r items from a set of n items. This concept is fundamental in probability and statistics, as it helps in calculating the likelihood of various outcomes.

Binomial Coefficient

The binomial coefficient, represented as nCr, is a mathematical expression that calculates the number of ways to choose r elements from a set of n elements without regard to the order of selection. It is calculated using the formula nCr = n! / (r!(n-r)!), where '!' denotes factorial, the product of all positive integers up to that number. This concept is crucial for solving problems involving combinations.

Factorial

A factorial, denoted as n!, is the product of all positive integers from 1 to n. Factorials are used in various mathematical calculations, particularly in permutations and combinations. For example, 5! = 5 × 4 × 3 × 2 × 1 = 120. Understanding factorials is essential for evaluating expressions involving combinations, as they form the basis of the calculations.