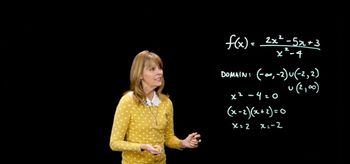Match the rational function in Column I with the appropriate descrip-tion in Column II. Choices in Column II can be used only once. ƒ(x)=(x^2-16)/(x+4)

Verified step by step guidance

1. <Factor the numerator of the rational function \( f(x) = \frac{x^2 - 16}{x + 4} \).>
2. <Recognize that \( x^2 - 16 \) is a difference of squares, which can be factored as \((x - 4)(x + 4)\).>
3. <Rewrite the function as \( f(x) = \frac{(x - 4)(x + 4)}{x + 4} \).>
4. <Identify that the \( x + 4 \) terms in the numerator and denominator can be canceled, but note the restriction that \( x \neq -4 \) to avoid division by zero.>
5. <Conclude that the simplified form of the function is \( f(x) = x - 4 \) for \( x \neq -4 \), and determine the type of discontinuity at \( x = -4 \).>

Key Concepts

Rational Functions

A rational function is a function that can be expressed as the quotient of two polynomials. In the given function ƒ(x)=(x^2-16)/(x+4), the numerator is a polynomial of degree 2, and the denominator is a polynomial of degree 1. Understanding the structure of rational functions is essential for analyzing their behavior, including identifying asymptotes and intercepts.

Factoring Polynomials

Factoring polynomials involves rewriting a polynomial as a product of its factors. For example, the numerator x^2-16 can be factored into (x-4)(x+4), which is a difference of squares. This process is crucial for simplifying rational functions and identifying points of discontinuity or removable discontinuities in the function.

Domain of a Function

The domain of a function refers to the set of all possible input values (x-values) for which the function is defined. For rational functions, the domain is restricted by values that make the denominator zero. In this case, since the denominator x+4 equals zero when x=-4, the domain of ƒ(x) excludes this value, impacting the function's overall behavior.