Here are the essential concepts you must grasp in order to answer the question correctly.
Polynomial Inequalities
Polynomial inequalities involve expressions where a polynomial is compared to a value using inequality signs (e.g., <, >, ≤, ≥). To solve these inequalities, one typically finds the roots of the polynomial, determines the intervals on the number line, and tests these intervals to see where the inequality holds true.
Recommended video:
Graphing Solution Sets
Graphing solution sets on a real number line visually represents the intervals where the inequality is satisfied. This involves marking the critical points (roots) and shading the appropriate regions based on whether the inequality is strict or inclusive, helping to clearly communicate the solution.
Recommended video:
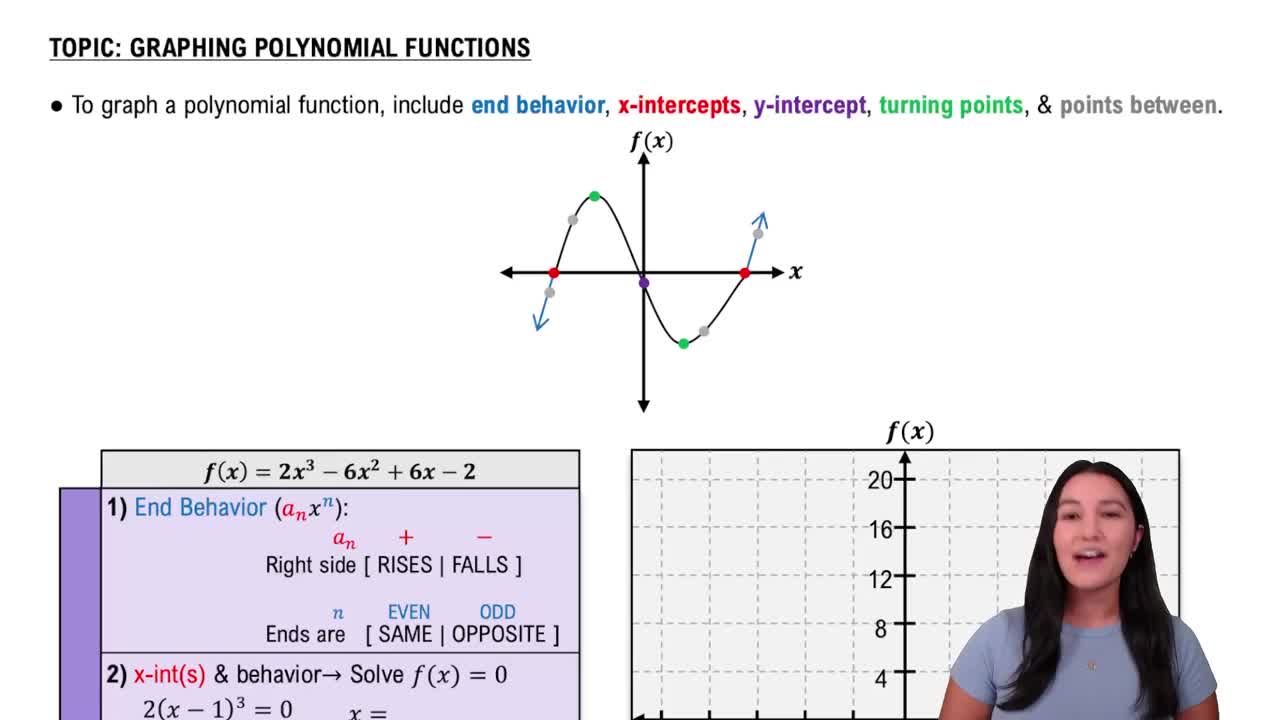
Graphing Polynomial Functions
Interval Notation
Interval notation is a mathematical notation used to represent a range of values. It uses parentheses and brackets to indicate whether endpoints are included (closed interval) or excluded (open interval). For example, (a, b) means all numbers between a and b, not including a and b, while [a, b] includes both endpoints.
Recommended video:
 Verified step by step guidance
Verified step by step guidance Verified Solution
Verified Solution