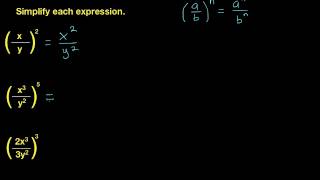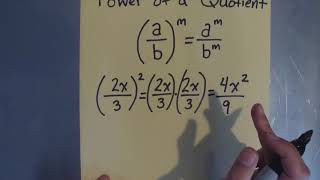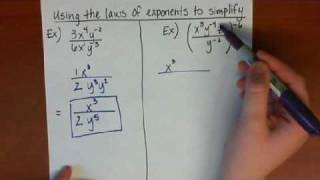Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
0. Review of Algebra
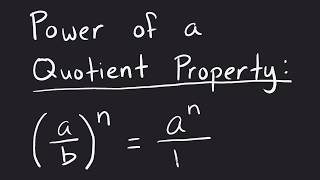
Exponents
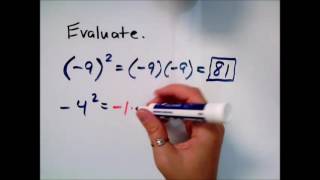
Problem 9c
Textbook Question
Find {16, 18, 21, 50} ∪ {15, 16, 17, 18}.
 Verified step by step guidance
Verified step by step guidance1
Identify the two sets involved in the union operation: Set A = \{16, 18, 21, 50\} and Set B = \{15, 16, 17, 18\}.
Understand that the union of two sets, denoted as A \cup B, is a set containing all distinct elements from both sets.
List all elements from both sets: 16, 18, 21, 50, 15, 17.
Remove any duplicate elements to ensure each element appears only once in the union.
Write the final set containing all unique elements from both sets.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
1mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Set Union
Set union is a fundamental operation in set theory that combines all unique elements from two or more sets. The union of sets A and B, denoted as A ∪ B, includes every element that is in A, in B, or in both. For example, if A = {1, 2} and B = {2, 3}, then A ∪ B = {1, 2, 3}.
Recommended video:

Finding the Domain and Range of a Graph
Elements of a Set
An element of a set is an individual object or number that belongs to that set. Sets are defined by their elements, which can be numbers, letters, or other sets. In the context of the question, the elements {16, 18, 21, 50} and {15, 16, 17, 18} are the distinct items that will be combined in the union operation.
Recommended video:
Graphing Polynomial Functions
Unique Elements
In set theory, a set is defined by its unique elements, meaning that duplicates are not counted. When performing operations like union, any repeated elements from the combined sets are included only once in the resulting set. For instance, in the union of {1, 2} and {2, 3}, the result is {1, 2, 3}, with '2' appearing only once.
Recommended video:
Guided course

Factor Using the AC Method When a Is 1

 7:39m
7:39mWatch next
Master Introduction to Exponent Rules with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice