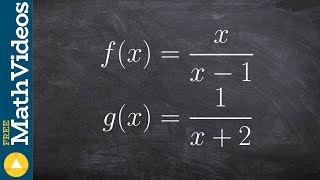Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
3. Functions
Function Composition
Problem 45c
Textbook Question
Use the definition of inverses to determine whether ƒ and g are inverses. f(x) = x^2+3, x≥0; g(x) = √x-3, x≥3
 Verified step by step guidance
Verified step by step guidance1
Step 1: To determine if two functions \( f \) and \( g \) are inverses, we need to check if \( f(g(x)) = x \) and \( g(f(x)) = x \).
Step 2: Start by finding \( f(g(x)) \). Substitute \( g(x) = \sqrt{x-3} \) into \( f(x) = x^2 + 3 \). This gives \( f(g(x)) = (\sqrt{x-3})^2 + 3 \).
Step 3: Simplify \( f(g(x)) \). The expression \( (\sqrt{x-3})^2 \) simplifies to \( x-3 \), so \( f(g(x)) = x-3 + 3 = x \).
Step 4: Now, find \( g(f(x)) \). Substitute \( f(x) = x^2 + 3 \) into \( g(x) = \sqrt{x-3} \). This gives \( g(f(x)) = \sqrt{(x^2 + 3) - 3} \).
Step 5: Simplify \( g(f(x)) \). The expression \( (x^2 + 3) - 3 \) simplifies to \( x^2 \), so \( g(f(x)) = \sqrt{x^2} = x \), given \( x \geq 0 \). Since both \( f(g(x)) = x \) and \( g(f(x)) = x \), \( f \) and \( g \) are inverses.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Inverse Functions
Inverse functions are pairs of functions that 'undo' each other. For functions f and g to be inverses, applying g to the output of f should return the original input, and vice versa. Mathematically, this means f(g(x)) = x and g(f(x)) = x for all x in the domain of the respective functions.
Recommended video:

Graphing Logarithmic Functions
Domain and Range
The domain of a function is the set of all possible input values (x-values) for which the function is defined, while the range is the set of all possible output values (y-values). When determining if two functions are inverses, it is crucial to consider their domains and ranges, as they must align appropriately for the inverse relationship to hold.
Recommended video:

Domain & Range of Transformed Functions
Composition of Functions
The composition of functions involves combining two functions to create a new function. For functions f and g, the composition f(g(x)) means substituting g(x) into f. To verify if f and g are inverses, we need to compute both f(g(x)) and g(f(x)) and check if both equal x, confirming their inverse relationship.
Recommended video:

Function Composition

 4:56m
4:56mWatch next
Master Function Composition with a bite sized video explanation from Nick Kaneko
Start learningRelated Videos
Related Practice