Here are the essential concepts you must grasp in order to answer the question correctly.
Factoring and Products of Binomials
Factoring involves breaking down expressions into simpler components, often binomials. The product of two binomials can be found using the distributive property or the FOIL method (First, Outside, Inside, Last). In this case, (3y-5)(3y+5) is a difference of squares, which simplifies to 9y^2 - 25.
Recommended video:
Factor Using Special Product Formulas
Difference of Squares
The difference of squares is a specific algebraic identity that states a² - b² = (a - b)(a + b). This identity is crucial for simplifying expressions like (3y-5)(3y+5), where a = 3y and b = 5. Recognizing this pattern allows for quick simplification to 9y² - 25.
Recommended video:
Solving Quadratic Equations by Completing the Square
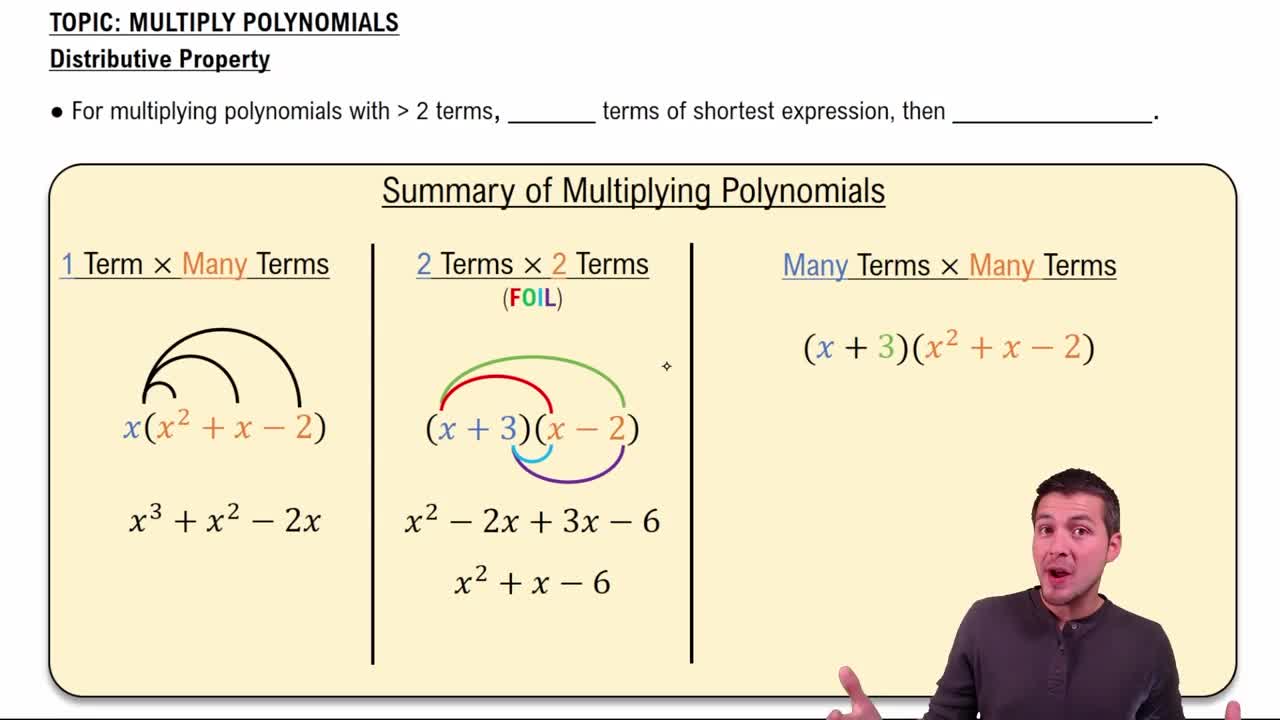
Multiplying Polynomials
Multiplying polynomials involves applying the distributive property to combine terms. When multiplying a binomial by a trinomial or another binomial, each term in the first polynomial must be multiplied by each term in the second. In this case, after simplifying (3y-5)(3y+5) to 9y² - 25, the next step is to multiply this result by any additional polynomials, if present.
Recommended video:
Multiply Polynomials Using the Distributive Property
 Verified Solution
Verified Solution


 5:13m
5:13m
