Here are the essential concepts you must grasp in order to answer the question correctly.
Polynomial Multiplication
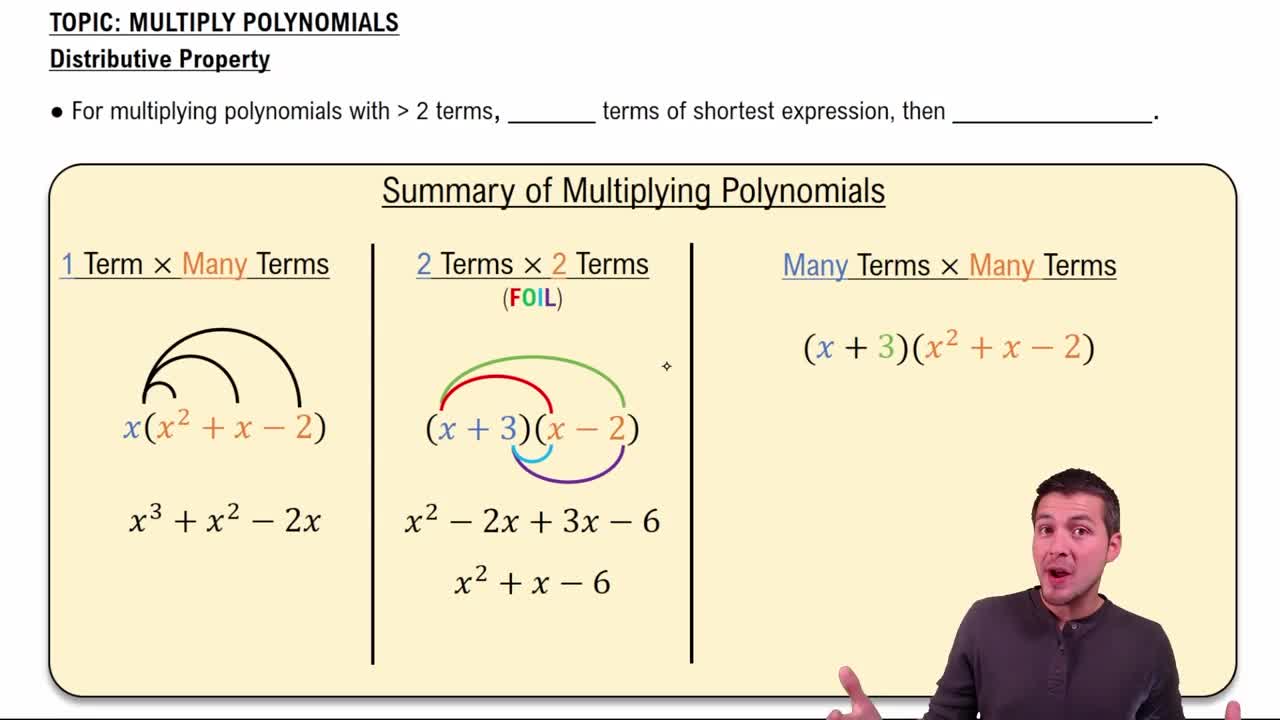
Polynomial multiplication involves distributing each term in one polynomial to every term in another polynomial. This process, often referred to as the distributive property, ensures that all combinations of terms are accounted for, leading to a new polynomial that combines like terms. For example, in the expression (7x^3 + 5)(x^2 - 2), each term in the first polynomial must be multiplied by each term in the second.
Recommended video:
Finding Zeros & Their Multiplicity
Distributive Property
The distributive property states that a(b + c) = ab + ac, allowing us to multiply a single term by a sum. This property is essential in polynomial multiplication, as it simplifies the process of expanding expressions. In the given problem, applying the distributive property helps in systematically multiplying 7x^3 and 5 with both terms in (x^2 - 2).
Recommended video:
Multiply Polynomials Using the Distributive Property
Combining Like Terms
Combining like terms is the process of simplifying an expression by adding or subtracting terms that have the same variable raised to the same power. After multiplying polynomials, the resulting expression may contain several terms that can be simplified. In the context of the problem, after performing the multiplication, it is crucial to identify and combine any like terms to arrive at the final simplified polynomial.
Recommended video:
 Verified step by step guidance
Verified step by step guidance Verified Solution
Verified Solution