Here are the essential concepts you must grasp in order to answer the question correctly.
Radical Expressions
Radical expressions involve roots, such as square roots, cube roots, etc. In this context, the expression √6 represents the square root of 6, which is a positive real number. Understanding how to manipulate these expressions is crucial for performing operations like addition, multiplication, and simplification.
Recommended video:
Radical Expressions with Fractions
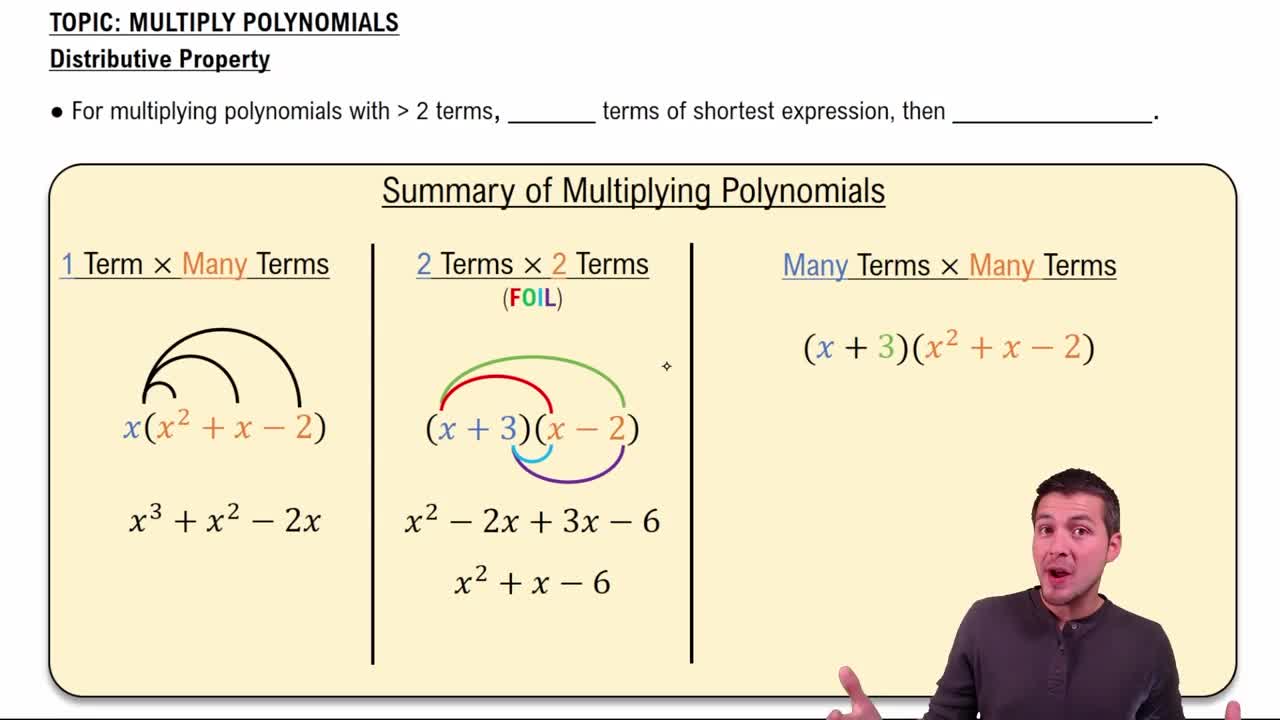
Distributive Property
The distributive property states that a(b + c) = ab + ac. This property allows us to multiply a single term by each term within a parenthesis. In the given expression, applying the distributive property will help in multiplying √6 by both terms inside the parentheses (3 and √7), leading to a simplified result.
Recommended video:
Multiply Polynomials Using the Distributive Property
Simplifying Radical Expressions
Simplifying radical expressions involves reducing them to their simplest form, which often includes combining like terms or rationalizing denominators. In this case, after applying the distributive property, it is important to simplify the resulting terms, especially when dealing with products of radicals, to ensure the final expression is as concise as possible.
Recommended video:
Radical Expressions with Fractions
 Verified step by step guidance
Verified step by step guidance Verified Solution
Verified Solution