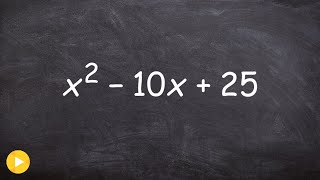In Exercises 1–30, factor each trinomial, or state that the trinomial is prime. Check each factorization using FOIL multiplication. x² − x + 7

Verified step by step guidance

1. Identify the trinomial: \(x^2 - x + 7\).
2. Check if the trinomial can be factored by looking for two numbers that multiply to the constant term (7) and add to the linear coefficient (-1).
3. Since 7 is a prime number, the only integer factors are 1 and 7, which do not add up to -1.
4. Conclude that the trinomial \(x^2 - x + 7\) cannot be factored using integer coefficients.
5. State that the trinomial is prime because it cannot be factored further over the integers.

Key Concepts

Factoring Trinomials

Factoring trinomials involves rewriting a quadratic expression in the form ax² + bx + c as a product of two binomials. This process requires identifying two numbers that multiply to ac (the product of a and c) and add to b. If such numbers do not exist, the trinomial is considered prime, meaning it cannot be factored over the integers.

Prime Trinomials

A prime trinomial is a quadratic expression that cannot be factored into simpler binomial expressions with integer coefficients. This occurs when the discriminant (b² - 4ac) is negative or when no integer pairs satisfy the conditions for factoring. Recognizing prime trinomials is essential for determining the limits of factorization.

FOIL Method

The FOIL method is a technique used to multiply two binomials, standing for First, Outside, Inside, Last, which refers to the order in which the terms are multiplied. This method helps verify the correctness of a factorization by ensuring that the product of the binomials returns to the original trinomial. It is a crucial step in confirming the accuracy of the factorization process.