In Exercises 73–74, use the graph of the rational function to solve each inequality.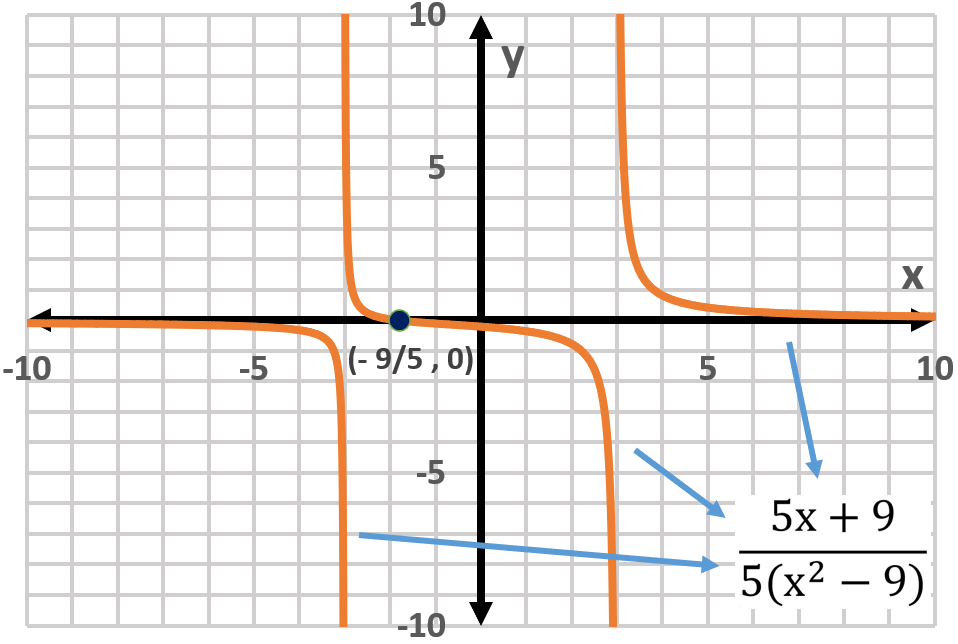
1/4(x + 2) > - 3/4(x - 2)

Verified step by step guidance

1. Step 1: Identify the rational function from the graph. The function given is \( \frac{x + 1}{x^2 - 4} \).
2. Step 2: Determine the vertical asymptotes by setting the denominator equal to zero and solving for x. This gives us \( x^2 - 4 = 0 \), which factors to \( (x - 2)(x + 2) = 0 \). Therefore, the vertical asymptotes are at \( x = 2 \) and \( x = -2 \).
3. Step 3: Determine the horizontal asymptote by comparing the degrees of the numerator and the denominator. Since the degree of the denominator (2) is greater than the degree of the numerator (1), the horizontal asymptote is at \( y = 0 \).
4. Step 4: Identify the x-intercept by setting the numerator equal to zero and solving for x. This gives us \( x + 1 = 0 \), so the x-intercept is at \( x = -1 \).
5. Step 5: Identify the y-intercept by evaluating the function at \( x = 0 \). This gives us \( f(0) = \frac{0 + 1}{0^2 - 4} = \frac{1}{-4} = -\frac{1}{4} \). So, the y-intercept is at \( y = -\frac{1}{4} \).