Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
7. Systems of Equations & Matrices
Two Variable Systems of Linear Equations
Problem 62
Textbook Question
Solve each problem. Find all values of b such that the straight line 3x - y = b touches the circle x^2 + y^2 = 25 at only one point.
 Verified step by step guidance
Verified step by step guidance1
Recognize that the line 3x - y = b is tangent to the circle x^2 + y^2 = 25 if it touches the circle at exactly one point.
Recall that the condition for tangency between a line and a circle is that the perpendicular distance from the center of the circle to the line equals the radius of the circle.
The center of the circle x^2 + y^2 = 25 is (0, 0) and the radius is 5.
Use the formula for the distance from a point (x_1, y_1) to a line Ax + By + C = 0, which is |Ax_1 + By_1 + C| / sqrt(A^2 + B^2).
Substitute the center (0, 0) into the distance formula for the line 3x - y = b, and set the distance equal to the radius 5 to find the values of b.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
9mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Circle Equation
The equation of a circle in standard form is given by (x - h)² + (y - k)² = r², where (h, k) is the center and r is the radius. In this case, the circle is defined by x² + y² = 25, indicating a center at (0, 0) and a radius of 5. Understanding this helps in visualizing the geometric relationship between the line and the circle.
Recommended video:

Circles in Standard Form
Line Equation
The equation of a line can be expressed in slope-intercept form as y = mx + b, where m is the slope and b is the y-intercept. The given line equation, 3x - y = b, can be rearranged to find its slope and intercepts. This is crucial for determining how the line interacts with the circle.
Recommended video:
Guided course
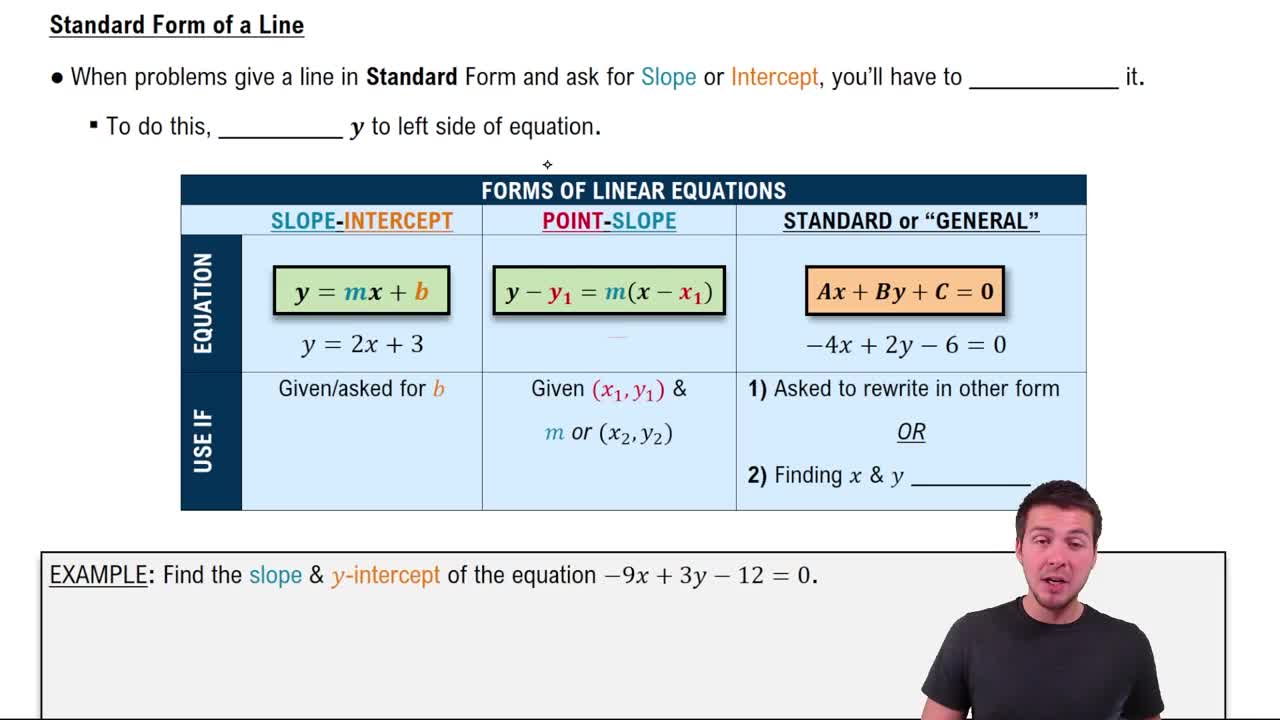
Standard Form of Line Equations
Tangency Condition
For a line to touch a circle at exactly one point, the distance from the center of the circle to the line must equal the radius of the circle. This condition can be mathematically expressed using the formula for the distance from a point to a line. Solving for b under this condition will yield the values where the line is tangent to the circle.
Recommended video:

Categorizing Linear Equations

 4:27m
4:27mWatch next
Master Introduction to Systems of Linear Equations with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice













