Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
1. Equations & Inequalities
Powers of i
Problem 12
Textbook Question
Identify each number as real, complex, pure imaginary, or nonreal com-plex. (More than one of these descriptions will apply.) 0
 Verified step by step guidance
Verified step by step guidance1
Step 1: Understand the types of numbers involved. Real numbers include all the numbers on the number line, complex numbers are of the form a + bi where a and b are real numbers, and pure imaginary numbers are of the form 0 + bi.
Step 2: Recognize that the number 0 can be expressed as 0 + 0i, which fits the form of a complex number where both the real part (a) and the imaginary part (b) are zero.
Step 3: Since 0 can be expressed as 0 + 0i, it is a complex number.
Step 4: The number 0 is also a real number because it is located on the real number line.
Step 5: Conclude that the number 0 is both a real number and a complex number.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
32sPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Real Numbers
Real numbers include all the numbers that can be found on the number line, encompassing both rational numbers (like integers and fractions) and irrational numbers (like √2 and π). They can be positive, negative, or zero, and they do not involve imaginary components.
Recommended video:

Introduction to Complex Numbers
Complex Numbers
Complex numbers are numbers that have a real part and an imaginary part, expressed in the form a + bi, where 'a' is the real part, 'b' is the coefficient of the imaginary unit 'i' (where i² = -1). They extend the concept of one-dimensional number lines to two dimensions, allowing for solutions to equations that have no real solutions.
Recommended video:

Dividing Complex Numbers
Imaginary Numbers
Imaginary numbers are defined as multiples of the imaginary unit 'i', which is the square root of -1. A pure imaginary number has no real part and is expressed as bi, where b is a real number. For example, 3i is a pure imaginary number, while 0 can be considered both real and imaginary since it can be expressed as 0 + 0i.
Recommended video:
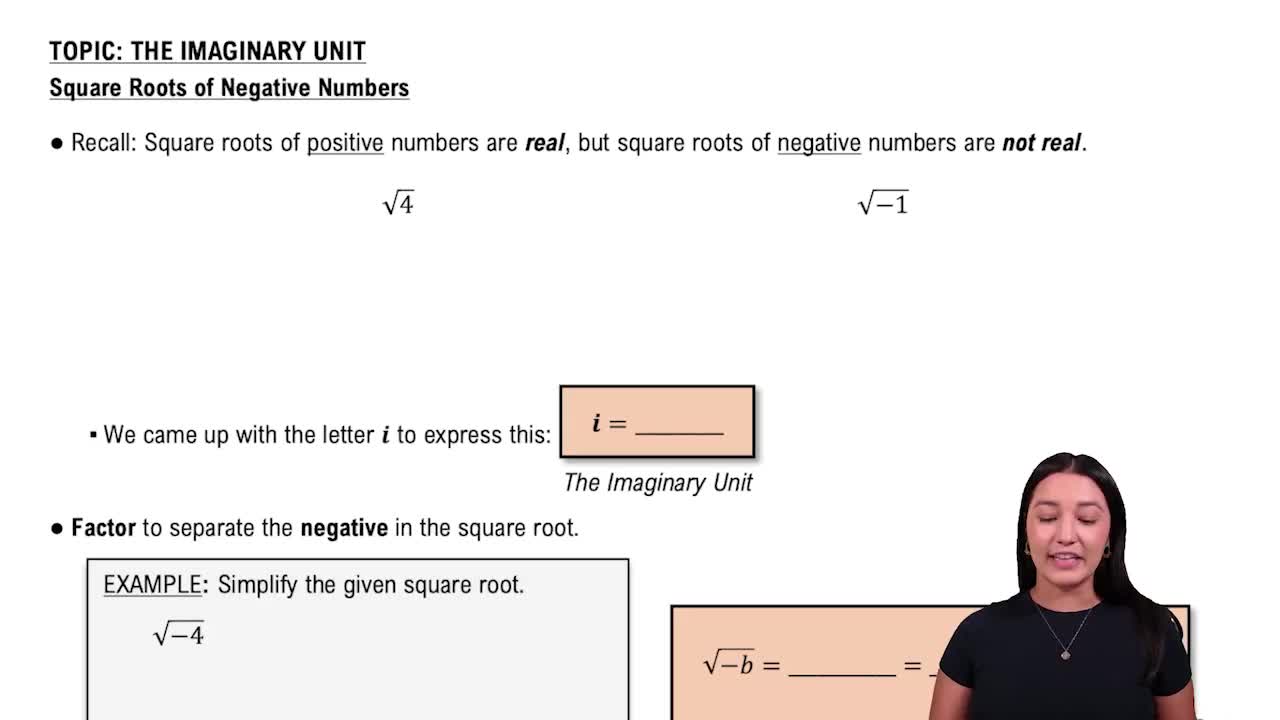
Square Roots of Negative Numbers
Related Videos
Related Practice






