Here are the essential concepts you must grasp in order to answer the question correctly.
Imaginary Numbers
Imaginary numbers are defined as multiples of the imaginary unit 'i', where i is the square root of -1. They arise when taking the square root of negative numbers, which do not have real solutions. For example, √-1 = i, and thus √-49 can be expressed as √(49) * √(-1) = 7i.
Recommended video:
Square Roots of Negative Numbers
Standard Form of Complex Numbers
The standard form of a complex number is expressed as a + bi, where 'a' is the real part and 'b' is the imaginary part. In the context of simplifying expressions involving square roots of negative numbers, it is essential to represent the result in this format. For √-49, the standard form would be 0 + 7i, simplifying to just 7i.
Recommended video:
Multiplying Complex Numbers
Properties of Square Roots
The properties of square roots state that √(a * b) = √a * √b and √(a/b) = √a / √b, applicable for non-negative 'a' and 'b'. When dealing with negative numbers, it is crucial to separate the real and imaginary components. This property allows us to break down √-49 into √49 and √-1, facilitating the simplification process.
Recommended video:
Imaginary Roots with the Square Root Property
 Verified step by step guidance
Verified step by step guidance Verified Solution
Verified Solution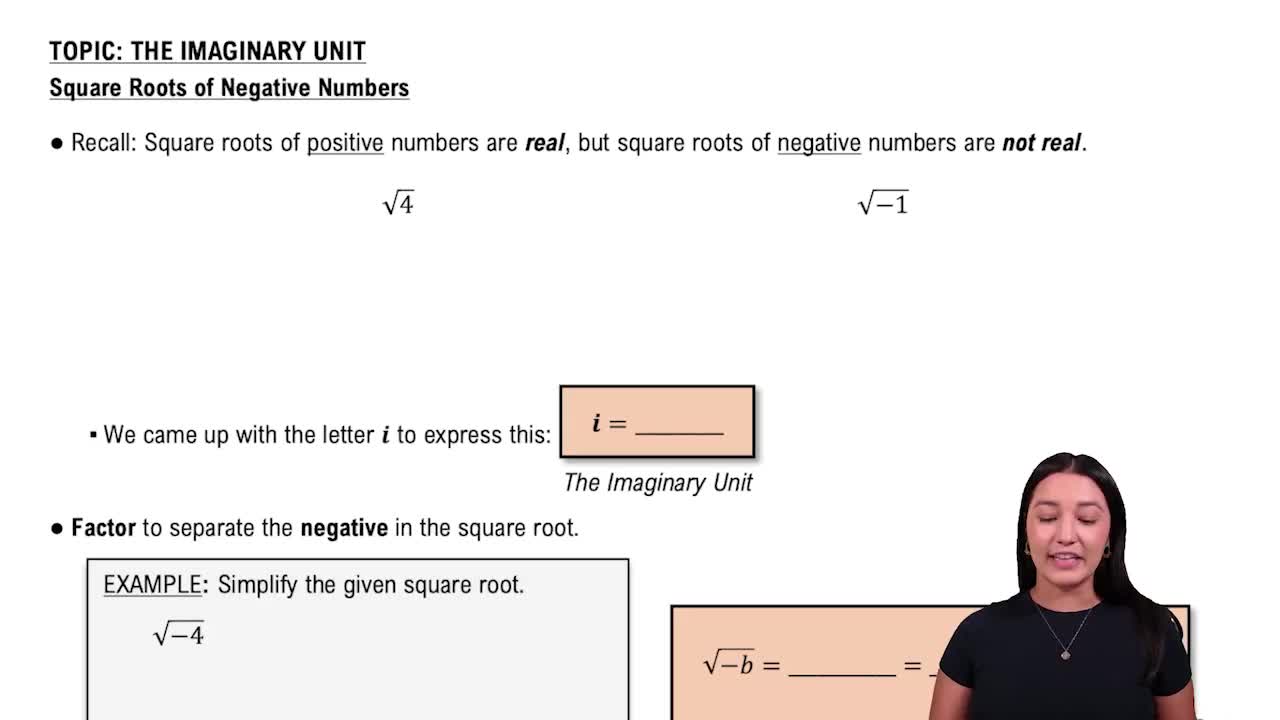



 5:02m
5:02m