Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
1. Equations & Inequalities
Rational Equations
Problem 57
Textbook Question
Solve each equation for x. 3x=(2x-1)(m+4)
 Verified step by step guidance
Verified step by step guidance1
Start by expanding the right side of the equation: \((2x - 1)(m + 4)\). Use the distributive property to expand it.
Distribute \((m + 4)\) to both terms inside the parenthesis: \(2x(m + 4) - 1(m + 4)\).
Simplify the expression: \(2xm + 8x - m - 4\).
Set the equation equal to the left side: \(3x = 2xm + 8x - m - 4\).
Rearrange the equation to isolate \(x\) on one side. Combine like terms and factor out \(x\) if possible.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Linear Equations
A linear equation is an algebraic equation in which each term is either a constant or the product of a constant and a single variable. The general form is ax + b = 0, where a and b are constants. Solving linear equations involves isolating the variable on one side of the equation to find its value.
Recommended video:

Categorizing Linear Equations
Distributive Property
The distributive property states that a(b + c) = ab + ac, allowing us to multiply a single term by two or more terms inside parentheses. This property is essential for simplifying expressions and solving equations, as it helps to eliminate parentheses and combine like terms effectively.
Recommended video:
Guided course
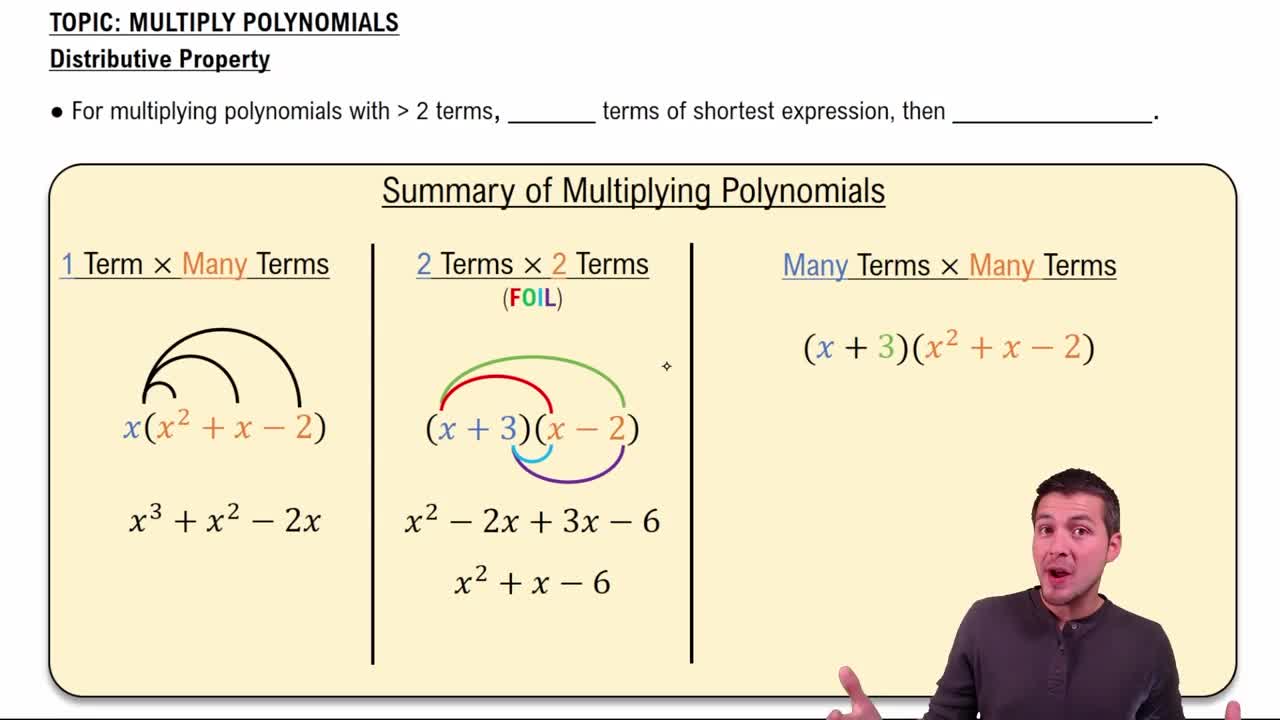
Multiply Polynomials Using the Distributive Property
Isolating the Variable
Isolating the variable is a key step in solving equations, where the goal is to get the variable (in this case, x) alone on one side of the equation. This often involves performing inverse operations, such as addition, subtraction, multiplication, or division, to both sides of the equation to maintain equality.
Recommended video:
Guided course

Equations with Two Variables

 5:56m
5:56mWatch next
Master Introduction to Rational Equations with a bite sized video explanation from Callie
Start learning


