Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
8. Conic Sections
Ellipses: Standard Form
Problem 67
Textbook Question
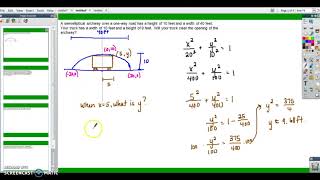
In Exercises 67–68, graph each semiellipse. y = √16 - 4x²
 Verified step by step guidance
Verified step by step guidance1
Identify the equation of the semiellipse: \( y = \sqrt{16 - 4x^2} \). This represents the upper half of an ellipse centered at the origin.
Rewrite the equation in the standard form of an ellipse: \( \frac{x^2}{4} + \frac{y^2}{16} = 1 \). This helps in identifying the semi-major and semi-minor axes.
Determine the semi-major and semi-minor axes: The semi-major axis is along the y-axis with length 4, and the semi-minor axis is along the x-axis with length 2.
Plot the vertices of the semiellipse: The vertices are at (0, 4) and (0, 0) along the y-axis, and (-2, 0) and (2, 0) along the x-axis.
Sketch the semiellipse: Draw a smooth curve connecting the vertices, forming the upper half of the ellipse.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
5mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Semiellipse
A semiellipse is a half of an ellipse, typically defined by its major and minor axes. In the context of the equation y = √(16 - 4x²), the semiellipse opens upwards, representing the positive square root. Understanding the shape and orientation of a semiellipse is crucial for graphing it accurately.
Graphing Quadratic Functions
The equation y = √(16 - 4x²) can be derived from a quadratic function, specifically in the form of y² = 16 - 4x². This relationship indicates that the graph is a conic section, and recognizing how to manipulate and graph quadratic functions is essential for visualizing the semiellipse.
Recommended video:

Graphs of Logarithmic Functions
Intercepts and Symmetry
Finding the intercepts of the graph is vital for sketching it accurately. The semiellipse will intersect the y-axis at (0, 4) and the x-axis at points where y = 0. Additionally, the symmetry of the graph about the y-axis simplifies the graphing process, as one can reflect points across the y-axis to complete the shape.
Recommended video:
Guided course

Graphing Intercepts

 5:12m
5:12mWatch next
Master Graph Ellipses at Origin with a bite sized video explanation from Nick Kaneko
Start learning