Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
0. Review of Algebra
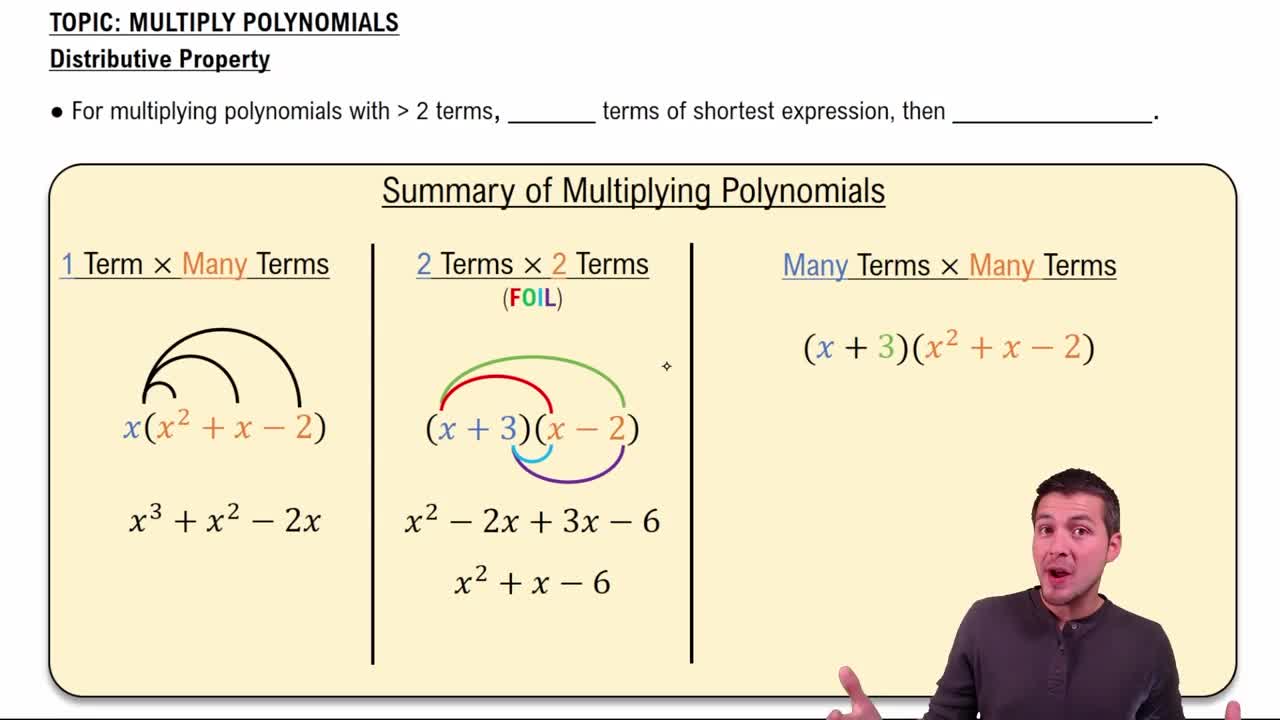
Multiplying Polynomials
Problem 60
Textbook Question
Find each product. See Examples 5 and 6. (r+3)^4
 Verified step by step guidance
Verified step by step guidance1
Recognize that \((r+3)^4\) is a binomial raised to a power, which can be expanded using the Binomial Theorem.
The Binomial Theorem states that \((a+b)^n = \sum_{k=0}^{n} \binom{n}{k} a^{n-k} b^k\). Here, \(a = r\), \(b = 3\), and \(n = 4\).
Calculate each term of the expansion using the formula: \(\binom{4}{k} r^{4-k} 3^k\) for \(k = 0, 1, 2, 3, 4\).
Compute the binomial coefficients \(\binom{4}{0}, \binom{4}{1}, \binom{4}{2}, \binom{4}{3}, \binom{4}{4}\) using the formula \(\binom{n}{k} = \frac{n!}{k!(n-k)!}\).
Combine all the terms to write the expanded form of \((r+3)^4\) by substituting the calculated coefficients and powers of \(r\) and \(3\).
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
4mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Binomial Theorem
The Binomial Theorem provides a formula for expanding expressions of the form (a + b)^n, where n is a non-negative integer. It states that (a + b)^n can be expressed as the sum of terms involving binomial coefficients, which represent the number of ways to choose elements from a set. This theorem is essential for expanding polynomials like (r + 3)^4 efficiently.
Recommended video:
Guided course

Special Products - Cube Formulas
Binomial Coefficients
Binomial coefficients are the numerical factors that appear in the expansion of a binomial expression. They are denoted as C(n, k) or 'n choose k', representing the number of ways to choose k elements from a set of n elements. In the context of the expansion of (r + 3)^4, these coefficients determine the weight of each term in the expansion, calculated using the formula C(n, k) = n! / (k!(n-k)!).
Recommended video:
Guided course

Special Products - Cube Formulas
Polynomial Expansion
Polynomial expansion is the process of expressing a polynomial in a simplified form by distributing and combining like terms. In the case of (r + 3)^4, this involves applying the Binomial Theorem to generate all terms of the polynomial, which will include powers of r and constants. Understanding how to expand polynomials is crucial for solving algebraic equations and simplifying expressions.
Recommended video:
Guided course

Introduction to Polynomials
Related Videos
Related Practice