Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
7. Systems of Equations & Matrices
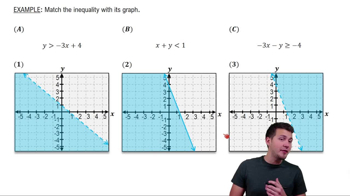
Graphing Systems of Inequalities
Problem 79
Textbook Question
Textbook QuestionFind the maximum and minimum values of each objective function over the region of feasible solutions shown at the right. objective function = 3x + 5y
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
3mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Objective Function
An objective function is a mathematical expression that defines the goal of an optimization problem, typically in the form of a linear equation. In this case, the objective function is 3x + 5y, which we aim to maximize or minimize. Understanding how to interpret and manipulate this function is crucial for determining optimal values of the variables involved.
Recommended video:
Permutations of Non-Distinct Objects
Feasible Region
The feasible region is the set of all possible points that satisfy the constraints of an optimization problem. It is typically represented graphically as a polygon on a coordinate plane. Identifying this region is essential because the maximum and minimum values of the objective function can only occur at the vertices of this region.
Recommended video:

Probability of Non-Mutually Exclusive Events Example
Linear Programming
Linear programming is a method used to achieve the best outcome in a mathematical model whose requirements are represented by linear relationships. It involves maximizing or minimizing a linear objective function subject to linear constraints. Familiarity with linear programming techniques, such as the graphical method, is necessary for solving the problem effectively.
Recommended video:

Linear Inequalities

 7:2m
7:2mWatch next
Master Linear Inequalities with a bite sized video explanation from Patrick Ford
Start learning