Here are the essential concepts you must grasp in order to answer the question correctly.
Complex Numbers
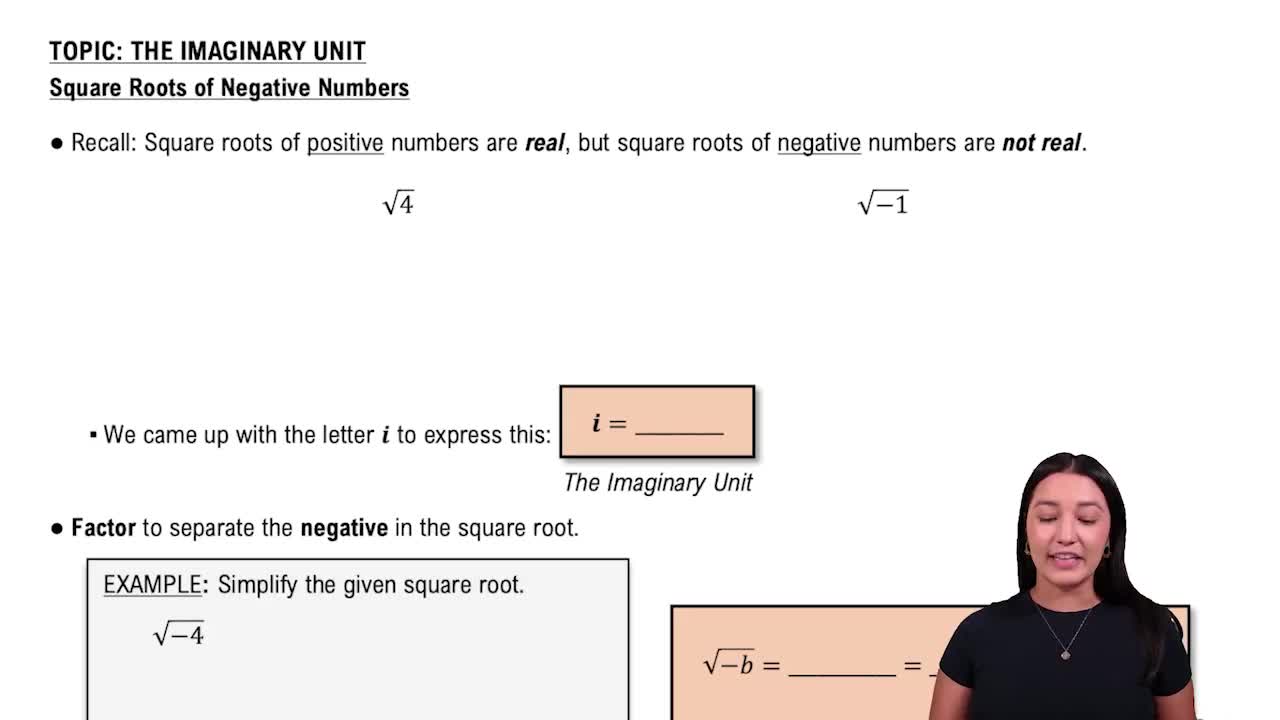
Complex numbers are numbers that have a real part and an imaginary part, expressed in the form a + bi, where 'a' is the real part and 'b' is the coefficient of the imaginary unit 'i', which is defined as the square root of -1. Understanding complex numbers is essential for performing operations involving square roots of negative numbers, as they allow us to extend the number system beyond real numbers.
Recommended video:
Square Roots of Negative Numbers
The square root of a negative number is not defined within the set of real numbers, but it can be expressed using complex numbers. For example, √-64 can be simplified to 8i, since √-64 = √(64) * √(-1) = 8 * i. This concept is crucial for solving problems that involve square roots of negative values, as it leads to the use of imaginary numbers.
Recommended video:
Square Roots of Negative Numbers
Standard Form of Complex Numbers
The standard form of a complex number is typically written as a + bi, where 'a' and 'b' are real numbers. When performing operations with complex numbers, such as addition or subtraction, it is important to combine like terms to express the result in this standard form. This ensures clarity and consistency in mathematical communication, especially when dealing with multiple complex numbers.
Recommended video:
Multiplying Complex Numbers
 Verified step by step guidance
Verified step by step guidance Verified Solution
Verified Solution